PRE2019 1 Group2

- Group -

• Kasper Dols - 0953689
• Marco Luijten - 1008931
• Wouter Meekes - 1011988

Main tutor: Tijn Borghuis

• Week 1
• Week 2
• Week 3
• Week 4
• Week 5
• Week 6

Mission to Europa

Introduction

Europa is a very interesting, mysterious moon of Jupiter, discovered by Galileo Galilei in the year 1610. The moon raised a lot of interest in the past couple of decades, because there are some indications of liquid water on the moon. Since water is at the top of the list of ingredients that make life possible, the speculations for extraterrestrial life on Europa began to rise. Water dissolves nutrients for organisms to eat, transports important chemicals within living cells and allows those cells to get rid of waste. [1] But due to the circumstances at Europa, the water is believed to be hidden underneath a thick coat of ice. This coat is estimated to be 10 kilometers around the whole moon, with a deviation of 160. [2] Calculations will be performed using this 10 km; the 160 m deviation will be considered negligible with respect to 10 km.

Presence of liquid water on the surface

But can there be water present in liquid form somewhere at the surface of Europa? Probably not. One of the reasons to assume this, is based on the phase diagram of water, shown to the right. As can be seen in the image, the lowest pressure at which water can still exist in liquid form is its triple point at 611.73 Pa (0.0061 atm), at the usual temperature of 273.15 K (0 °C). Below that pressure, water has no liquid form. Since the pressure at Europa’s surface is about 10^{-10} atm, this means that liquid water can not stably exist on the surface of Europa. Some water may come to surface for a brief moment, but will almost instantaneously either freeze or boil, leaving no water remaining. It should be noted that indeed this diagram does not extend below 10^-5 atm, and that based on this image it is thus technically not possible to say that water does not have a liquid form at such ultra-low pressures. However, it is first of all unlikely that such an out-of-place phase change exists based on this and other phase diagrams. Secondly, this ‘liquid’ may not be liquid as we know it and still be unable to support life. Much like solid water has different crystalline structures at different temperatures and pressures, so can this liquid water have very different properties based on the environment it is in. Hence based purely on physical grounds it is unlikely that liquid water in a familiar form exists on the surface of Europa. [3]

Presence of a sub-surface ocean

Why do researchers believe there is an sub-surface ocean? The first theories that the planet has a sub-surface ocean came after the fly-by mission of Voyager 1. This spacecraft was, in march 1979, the first spacecraft that made images in significant detail of Europa’s surface, with a resolution of about 2 kilometers per pixel. These images revealed a surprisingly smooth surface, brighter than that of earth’s moon, crisscrossed with numerous bands and ridges. Researchers noted that some of the dark bands had opposite sides that matched extremely well, comparable to pieces of a jigsaw puzzle. These cracks had separated, and dark, icy material appeared to have flowed into the opened gaps, suggesting that the surface had been active at some time in the past. The images also showed only a handful of big craters, which are expected to build up over billions of years as the planetary surface is bombarded by meteorites, until the surface is covered in craters. Thus, a lack of much craters suggested that Europa’s surface was relatively young and implied that something erased the craters, such as icy, volcanic flows. Next to that, scientists found patterns of some of the longest linear features in the images that did not match the predicted patterns of the features, created by tides as Europa orbits Jupiter. They determined that the found patterns would fit very well if Europa’s surface could move independently and was not locked to the rest of the interior. These interesting findings led to the next mission to Europa, Galileo. This spacecraft was launched in 1989 and entered orbit around Jupiter in 1995. Galileo eventually made 12 close flybys of the icy moon, including images of Europa at a range of scales, revealing new details about the surface and providing context for how those details were related to the moon as a whole. One important measurement made by the Galileo mission showed how Jupiter’s magnetic field was disrupted in the space around Europa, implying that a special type of magnetic field is being created within Europa by a deep layer of some electrically conductive fluid beneath the surface. Scientists believe, based on Europa’s icy composition, that the most likely material to create this magnetic signature is a global ocean of salty water. Above described are four strong indications of a sub-surface ocean on Europa, which is why the common belief under scientists is that the ocean really exists. [4]

Indications for life

The three basic requirements for life to be present are liquid water, chemical building blocks and a source of energy. The first requirement is explained in previous paragraph. The second requirement, the chemical building blocks, are also believed to be partly present. The ice and other materials on Europa’s surface are bombarded with radiation from Jupiter, that could alter them into some of the chemical building blocks of life, like oxygen (O₂), hydrogen peroxide (H₂O₂), carbon dioxide (CO₂) and sulfur dioxide (SO₂). If these compounds reach the sub-surface ocean, they can be valuable nutrients to start and sustain life. Besides, the ocean water can react with the rocks and minerals of the subsurface ocean’s floor to liberate other nutrients to support life. The third requirement is a source of energy. Europa’s position in space is within the powerful gravitational field of Jupiter, causing the moon into an orbit with one hemisphere constantly facing Jupiter. This elliptical orbit takes Europa alternatingly closer to and further away from Jupiter. This constant increase and decrease of gravitational force on Europa results in elongating and relaxing of the moon with each trip around the planet. This internal movement, combined with gravitational forces caused by neighboring moons, produces internal friction and heat within Europa. This internal heat could be the energy source that keeps the subsurface ocean from freezing and sustains any life that exists there. Next to that, there could be hot water vents on the floor of the subsurface ocean that deliver energy and nutrients from the planet’s interior. On earth, organisms have been discovered in the subglacial lakes of Antarctica and in hot ion-rich waters of hydrothermal vents. Life in Europa’s sub-surface ocean could be supported in a similar way. [5] These indications for life in Europa’s ocean have led to a future mission of NASA to the moon. They planned to launch the Europa Clipper mission in 2025. The spacecraft will conduct an in-depth exploration of Europa, investigating whether the moon could harbor conditions suitable for life. [6]

The goal

The above described mission of NASA is of course very interesting, but with the strong indications for life as described above, the interest rises to search for life on the spot. Since the presence of liquid water at Europa’s surface is unlikely as explained, the goal of this project is as follows:

“Investigate whether it is possible to land on Europa, dig through the icy layer and send a submarine into the sub-surface ocean, to search for life, signs of life, or conditions that may support life in or on Europa.”

Users

The question who is helped by going to Europa starts by asking why anyone would want to go to Europa in the first place. Ultimately, the humanity wants to learn stuff. In particular, the search for life outside earth. This can teach the humanity about the origin of life, and help to answer the age-old question: “Is there other life in this universe?” The reason to go to Europa and not just any other satellite in the solar system (possibly much closer) is that Europa is very likely to contain liquid water, which is one of the prerequisites for biological life, like explained in the introduction. The first most obvious question to ask then: “Is there (the possibility of) life on Europa?” This is what the mission first and foremost should answer. Furthermore, knowledge about Europa can help to learn about other exoplanets. By comparing our long-distance observations of Europa to the on-site observations, long-distance observations of exoplanets can be translated to planetary conditions. This may allow a more accurate prediction whether an exoplanet may be habitable. Lastly, a mission to put a lander on a planetoid like Europa has never been undertaken, and hence going to Europa will be a proof of concept showing that it is possible to go such a hostile environment. This is convenient information for a possible similar mission to, for instance, Pluto or an exoplanet. In the end, it is unknown what will be found on Europa. Maybe it contains about as much life as the centre of the sun, maybe it will show that life would be possible but never sprung up, or perhaps it turns out that it is home of the Atlanteans, who sunk their city on earth when they found earth with its dense atmosphere and high temperatures would not make for a habitable colony. Either way, it is also important to take into account the lives that might be encountered on Europa. It would be a pity to find all new types of bacteria on Europa, only to kill them with a stowaway extremophile hidden on the lander.

The users can largely be divided into 4 categories:

• Those executing this and other missions (SA’s)
• Companies other than the space agencies aiding in the mission (external)
• Those processing and using the results (scientists)
• Those potentially found during the missions (life)

Since direct communication over this distance is not possible, SA’s will want to be able to send commands to the vehicle such as ‘Go there’ or ‘Investigate this’, which the vehicle will carry out autonomously. For ‘investigate this’-commands, the vehicle should be able to recognise ‘this’ (‘this’ being whatever object it was instructed to investigate) and know how to investigate ‘this’. In ‘Go there’-commands, the vehicle should be able to know where it is on Europa and where its destination is. Furthermore, it should travel the distance and avoid or clear any obstacles it may come across. Furthermore, SA’s will want to be kept up-to-date on how the vehicle is doing. It should be capable of sending status updates to mission control about its own state. Furthermore, if something is found to be wrong, an ability to repair the vehicle could possibly save the mission. This updating will also give information to people planning a similar mission, about the feasibility and problems that are yet to be overcome. To avoid having to restart the mission on a monthly basis to accomplish the mission goals, some longevity on the vehicle is required. Both the energy and durability should last for a minimum t.b.d. period of time.

NASA usually outsources the building of their vehicles to other companies. The main assumption will be made that if the build is paid for, a company will be willing to build it, given that the build is legal. The vehicle must be brought to Europa in the first place. For this, the legality constraint applies as well. Furthermore, these carriers will have a rocket with a limited capacity, which sets a strong constraint on the maximum volume of the vehicle.

Scientists will want information on Europa itself; the chemical makeup of the crust, the atmosphere and the subsurface ocean, and the terrain of the crust. They will also want information on whether life exists there and/or could exist. This information will also help in the search for other habitable planets. For instance, measurements of Europa’s atmospheric density are done in terms of the column density (which counts the number of particles in a column with a particular ground surface area reaching all the way up into space), rather than the density of the atmosphere at surface level. Now, with a lander, the density of the atmosphere at surface level can be determined. This will yield a comparison between column density and surface density, which can be used for estimating the surface density of exoplanets based purely on column density. This may in the long run allow to find new planets to colonise, to redistribute the human load on the earth.

In case there is sentient life on Europa, they will most likely want to not be massacred. (This is deduced from the simple fact that if they are a civilization that would - for whatever reason - like to be massacred, they would’ve massacred themselves already.) Some form of communication is required. Furthermore, mission command will want them not to destroy the vehicle. As the nature and customs of any life is virtually impossible to determine as they evolved completely independent of Earthly conditions, preparing for this is equally complicated.

The list of users is as follows:

• External
  • Carriers
  • Builders
• Space agencies
  • Vehicle operators
  • Executives for other missions
• Scientists
  • Astronomers
  • Biologists
  • Humanity/ sociologists
• Life
  • Civilizations

User Requirements

Requirements overview

This list indicates the outline of the 7 main requirements for this project. The users most needing that requirement to be met are written behind the requirement in brackets. Of course most users require all requirements to be met in some capacity. For instance, if it is impossible to transport the vehicle to Europa, there will be no research. In that sense one might argue that the scientists also want the vehicle to be carriable. However, to them it is presumably unimportant whether the vehicle is indeed carried to Europa or teleported.

1. Carriable (Carriers)
2. Buildable (Builders)
3. Controllable (Operators)
4. Lasting (Operators and Executives for other missions)
5. Gets info on Europa (Astronomers)
6. Gets info on livability of Europa (Biologists and Humanity)
7. Treats Europa and its life ethically (Life)

Requirements extensive

This list expands on the overview presented above.

External

• 1 Get to Europa
• 2 Build vehicle
  • 2.1 Assuming that the mission is paid for, the builders will build it, so long as it is legal

SA’s

• 3 Command vehicle
  • 3.1 Autonomous execution of following category of commands
    • 3.1.1 Go there
    • 3.1.2 Investigate this
• 4 Longevity
  • 4.1 Sufficient energy
  • 4.2 Sufficient durability

Scientists

• 5 Info on Europa
  • 5.1 Atmosphere
    • 5.1.1 Ionosphere; plasma density, magnetic field, current
    • 5.1.2 Temperature and pressure
  • 5.2 Subsurface ocean
    • 5.2.1 Density
    • 5.2.2 Viscosity
    • 5.2.3 Salinity
  • 5.3 Crust
    • 5.3.1 Terrain
  • 5.4 All environments
    • 5.4.1 Chemical makeup
• 6 Info on life
  • 6.1 Possibility
    • 6.1.1 Required chemicals
    • 6.1.2 Required environment
  • 6.2 Itself
    • 6.2.1 Chemical makeup
    • 6.2.2 Habitat (Link data of life to data of the habitat)
    • 6.2.3 Enzymes

Life

• 7 Do not eliminate
  • 7.1 Preserve habitat (as little perturbing as possible)
  • 7.2 Non-lethal research methods
  • 7.3 Sterilised equipment

User Preferences

These are the preferences based on what the different users would like, given unlimited resources.

• 4 Longevity
  • 4.3 Keep up-to-date on vehicle status
    • 4.3.1 Recognise and report on faulty equipment
    • 4.3.2 Possibly repair faulty equipment

• 6 Info on life
  • 6.2 Itself
    • 6.2.4 DNA
    • 6.2.5 Complexity

Constraints

These are the constraints resulting from the implications of the user requirements and preferences. For instance, 'surviving Europa' implies being able to operate at temperatures between 86 and 132 K.

External

• 1 Carriable by Falcon Heavy
  • 1.1 Must fit inside cylindrical capsule: (L=13.1 m, r=2.6 m)
  • 1.2 Must be under 3500 kg (possibly a bit more, but if at all it is negligible)
• 2 Must be legal

SA’s

• 3 Controllable
  • 3.1 Autonomous execution
    • 3.1.1 'Go there'
      • 3.1.1.1 Know current and destiny locations
      • 3.1.1.2 Move
      • 3.1.1.3 Recognise obstacles on the way
    • 3.1.2 Investigate this
      • 3.1.2.1 Recognise ‘this’
      • 3.1.2.2 Know how to and be able to investigate ‘this’ (possibly in the command)
• 4 Longevity

Things prone to wear and tear or able to run out should run for at least 12 years.

• • 4.1 operate for 12 years, Either:
    • 4.1.1 carry enough energy
    • 4.1.2 Produce energy there
  • 4.2 Durability
    • 4.2.1 Iono- & Magnetosphere

Magnetic fields estimated at 5.0*10^-7 T Electron densities of up to 10¹⁰ m^-3 with energies up to 250 eV Ionospheric currents up to .42 A/m[7]

• • • 4.2.2 Low gravity

Calculations of non-uniform gravity, suggestions for zero-G car [8]

• • • 4.2.3 Low atmospheric pressure

Oxygen densities of around 10^-10 that of earth (~1.801*10²³ cm^-2), calculation in the following sources[9] [10] [11] [12]

• • • 4.2.4 Low temperatures

86-132 K[13]

• • • 4.2.5 Possibly rough or slippery surface

Bases should be able to maintain their position on the surface

• • • 4.2.6 Withstand tectonic activity

Precise magnitude unknown

• • • 4.2.7 Withstand high oceanic pressures

Up to .3 GPa of pressure (Calculation)

Life

• 7 Do not kill
  • 7.1 Radioactive sources amply shielded
  • 7.2 Research methods that do not kill the subject
  • 7.3 No biological earthly life brought along on the mission

Measurability Requirements and Constraints

These are the conditions to be fulfilled in order for the main requirements to be met.

1. The requirement to actually get to Europa has been laid into the hands of SpaceX. Requirement 1 has been fulfilled if the total lander system fits inside a capsule with a length of 13.1 m and a radius of 2.6 m, and is no heavier than 3500 kg.
2. Requirement 2, which asks for the lander system to be built, is fulfilled if the lander system contains only technology which is legal right off the bat, or for which it is possible to get a permit.
3. The requirement of autonomous execution of commands will be fulfilled if the vehicle has systems in place that allow it to know where it is, where it should end up, and how to get there without colliding with obstacles. Furthermore, it should be able to receive commands to research the things specified under requirements 5 and 6, it should be able to find these things, and know how to research them.
4. Requirement of survival is met if the lander system carries enough energy to sustain itself for at least 2 years or can produce on the spot enough energy for that period of time. Furthermore, it should hold on for these five years under the following conditions:
   1. External magnetic field of up to 1 mT, 2000 times stronger than what is estimated for Europa’s magnetosphere. Furthermore, the lander system should hold up at plasma densities of up to 10¹⁰ m^-3.
   2. The lander system should still function at gravities down to 10% of earth’s and should be able to account for a non-uniform gravity not always perpendicular to the surface.
   3. The lander system can still operate under pressures down to 10^-10 atmospheres. At the same time, the digger must be able to withstand pressures of at least 105 atm, and preferably up to 3000 atm.
   4. Temperatures between 86 and 132 K do not damage the lander system and its components, nor make it thusly prone to damage that normal operation will result in damage to the system.
   5. System bases (components of the system that do not move around on Europa) should be able to maintain a fixed position on or in Europa.
   6. The lander system should not be destroyed by the tectonic activity of Europa.
   7. Preference for survival is met if the lander system can recognise faulty equipment and report on it, and can repair or replace said equipment.
5. Requirement of planet research will be met if the lander system can gather data on the aforementioned aspects of Europa.
6. Point a. can be deduced from requirement 5, but to that end point 5 should be expanded to include the criteria for life. Point b. will be met if the lander system can gather data on the aforementioned aspects of any life found on Europa.
7. The requirement of ethical treatment of life and its environment will be met if research can be conducted in such a way as to avoid unethical harm done to relevant moral agents.

The Plan

Figure 1: Overview of the entire Europa mission

Figure 2: 8 interesting points on Europa

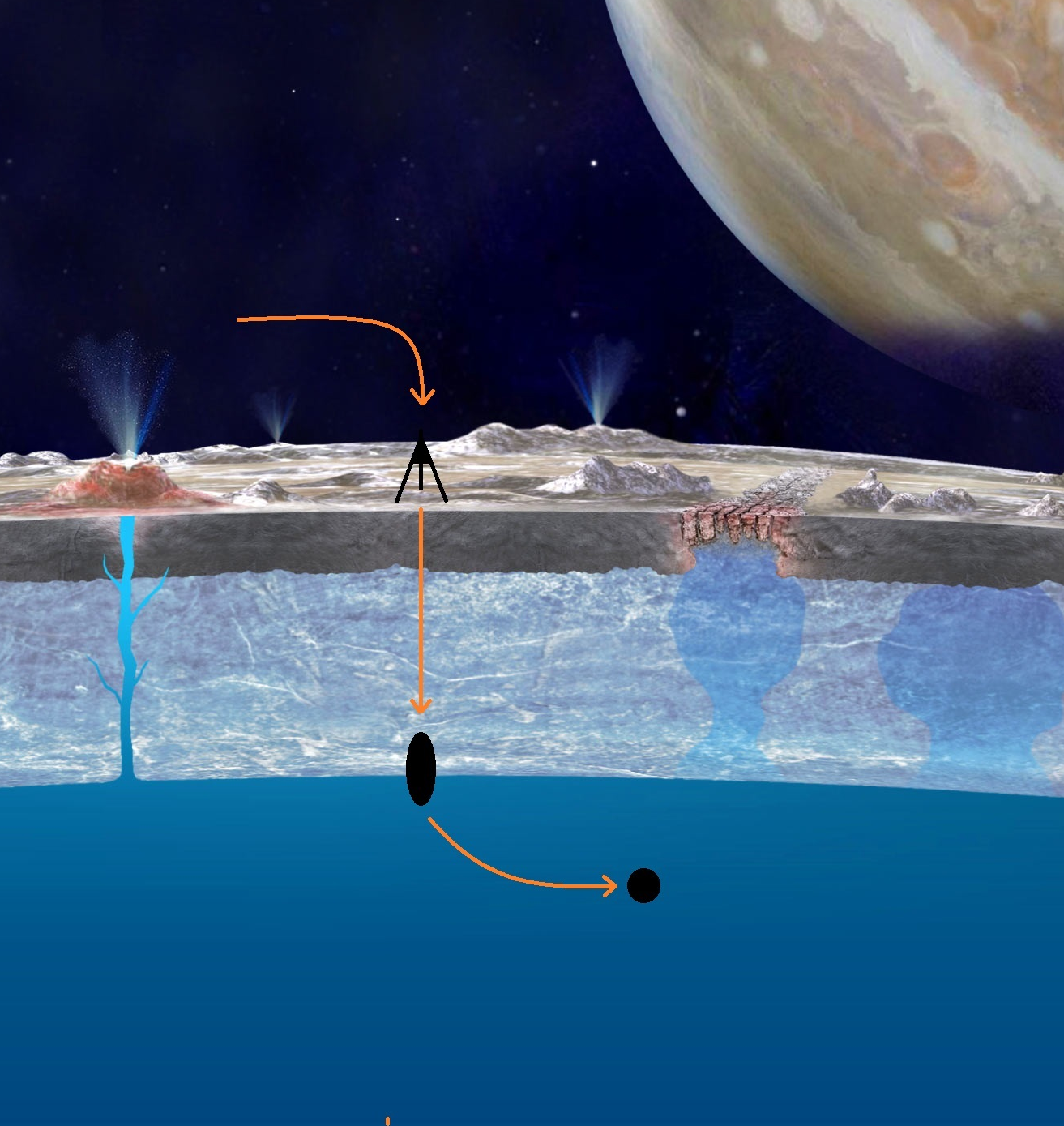
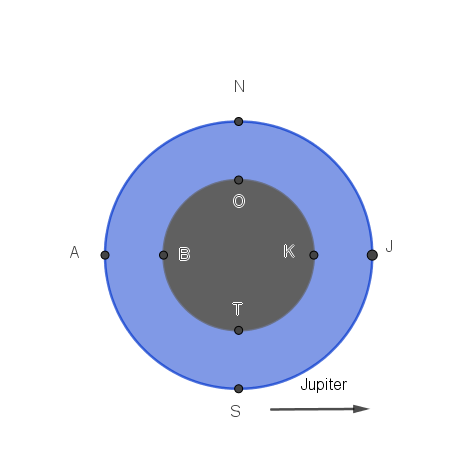
Before outlining the research and design in detail, a quick overview of the general mission will be presented here. This is also shown in the picture to the left. All this will be expanded on in the following chapters. First of all, a surface base will land on Europa’s crust. This base can conduct research at surface level, upholds contact with the earth. Furthermore, it holds a digger, that will begin to go through the crust right after the surface base has landed. Once the digger has breached through to the ocean, it will anchor itself in the ice and release a submarine which will do the bulk of the research. The digger maintains direct contact with the surface base. The submarine will sail through the ocean to find life, signs of life, or the possibility of life. It will do research in as large a range of circumstances as possible. The lower picture on the right shows 8 interesting points on Europa. The broadest range of circumstances is considered based on 2 parameters: Depth in the ocean (point A vs B or S vs T in the picture to the right) and proximity to Jupiter (A vs J). The latter difference is interesting because of the large impact that Jupiter has on Europa, in particular through tectonic heating. Thus, an optimal trajectory through Europa’s ocean would be ABOKJ or ABTKJ as seen in the picture to the right.

Performing Research

Experiments

Seismic experiments: A seismometer can be used by the station to determine the thickness of the ice of Europa’s crust, and possible determine a suitable entry point for the ice digger to start digging at. Furthermore, the seismic experiments may also result in observations about the size of the underwater ocean(s), giving scientists new data to correlate already made observations to. Since the lander will not be going under the ice, the seismic experiments will be performed solely on the surface. Similarly, since the station will not be changing its location on the surface, the seismic experiments will only be performed on the location that the surface lander lands on.

Spectrometry experiments: The submarine will carry a spectrometer to perform spectrometric experiments on the water below the ice. These experiments will show scientists the composition of the water, and maybe even the presence of life-sustaining molecules. The life-sustaining molecules that the submarine looks for belong to one of four categories:

• Carbohydrates, which supply an organism with energy and structure
• Lipids, which are organic molecules that consist of a hydrophobic and a hydrophilic side
• Proteins, which may serve a very wide array of functions within an organism
• Nucleic acids, which are molecules in an organism that carry information about said organism[14]

As for the location of performing spectrometric experiments, they will be performed at several locations. Since on Earth, different depths in the ocean harbor different forms of life, the mission probably has the highest chance of success if the search for life is performed at different depths, ranging from just below the ice to as deep as the submarine can go without being crushed.

Photos: Taking photos from the surface of Europa is not only cool, if the lander is equipped with a microscope or a likewise instrument, evidence or traces of life might be found, similar to how fossils and microscopic organisms on Earth provide evidence of past and present life. Of course, the surface is not the only thing that should be photographed. Having a camera on board of the submarine will enable photos of the ocean to also be taken. These photos could reveal the internal structure of Europa, or, in the very best case scenario, living organisms themselves in the ocean. Similar to the spectrometric experiments, the submarine will take photos at different depths, to maximize the chances of taking pictures of actual life. The submarine will start taking pictures just below the ice, and intermittently take new pictures as it reaches new depths.

Gas emission experiments: Similar to how the Viking landers performed gas release experiments on Mars, the same experiments could be performed on Europa. If a small sample of soil, or, in this case, water, is injected with radioactively labeled nutrients, the release of metabolised molecules may indicate that life is present. These gas emission experiments can not only be performed to find organisms that metabolise to CO2, it can also used to find organisms that metabolise CO2 itself, for example photosynthetic organisms. Once again, similar to the spectrometric experiments and the photos, the gas emission experiments will be performed at different depths, in hopes of obtaining evidence of the presence of life in Europa’s ocean. At these different depths, different organisms may be found, maximizing the chances of successful execution of the mission.

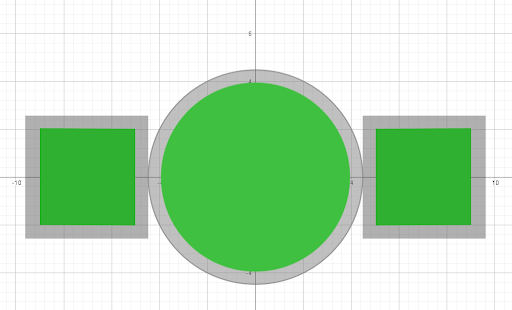
How to let water in and out of the submarine

Figure 3: Sketch of the system

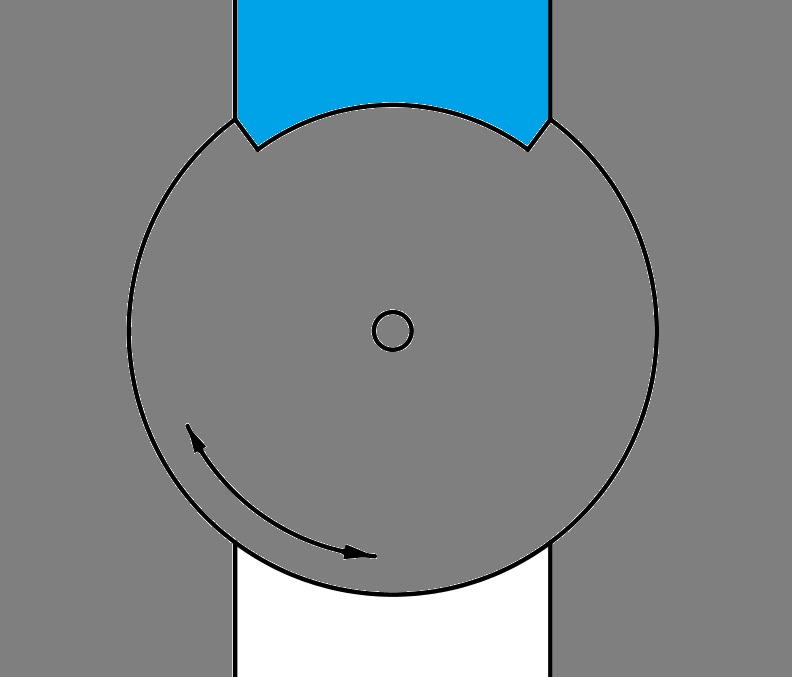
To perform the spectrometric experiments and the gas emission experiments, a sample of water will have to be let into the submarine, so the internal spectrometer can evaluate this sample. Just below the ice, this will not be a problem, but the deeper the submarine goes, the more pressure the water will put on it. These pressures will become very high, up to .3 GPa. The main problem when letting water in and out is to overcome the .3 GPa of pressure without having to do too much work, as this work requires energy that is probably not available. To achieve this, a rotating disk with a small slot for sampling water is proposed, as shown in figure 3. This disk will be encapsulated in the hull of the submarine, with sufficient strengthening around it to compensate for the puncturing of the hull. As the disk rotates, it will take with it a volume of water equal to the volume missing from the full disk. After half a rotation, it will deposit the water on the inside where it can flow to where it needs to be sampled via a simple tube. A disadvantage is that the submarine needs a particular orientation for the water to be able to fall in or out of the slot. However, being a submarine this should not be a problem. The volume of water sampled is equal to t*a*(R²-(R-d)²), where t is the disk thickness, a the angle that the slot spans in radians, R the outer radius of the disk and d the depth of the slot. For instance, at t=.3 cm, R=14 cm and d=2 cm, a needs only be 1.28 radians (73.4 degrees) for the slot to contain 10 mL. This sample size is based on one of the volumes that a cuvette of a spectrometer normally has, 1 mL. Given that 2 different experiments are performed, this gives a sample size of 2 * 1 mL = 2 mL. If, then, a margin of safety is added to this sample size, to account for any loss of sample size during intake, an intake volume of 10 mL would be a safe sample size to perform the experiments on. The biggest advantage of this system is that because the water is allowed to push on both sides of the slot in the disk, it attempts to push the disk both forward and backward with equal force, resulting in a net force due to water pressure of 0 N. By comparison, the total torque applied on either side of the slot at 150 km depth with the above parameters is 4680 Nm. By comparison, the SSC Tuatara‘Hypercar’ caps out at a meagre 1735 Nm of torque, and it has a motor weighing nearly 200 kg.[15] This goes to show the significant advantage of using the rotating slot. Of course it will still require some force, as the disk needs to rotate without gaps for the water to seep through. Hence both the disk and the casing around it need to be extremely smooth to decrease the coefficient of friction as much as possible. Hwang and Zum Gahr found the friction coefficient between 2 plates of polished steel to be about 0.116.[16] With a slot depth of 6 cm and volume of 10 ml, this means that as the water pushes down on the disk through the opening - 7 cm wide - with a total force of .3*10⁹*.003*.07=62962 N, the friction force to be overcome is 7304 N, resulting in a torque of 1023 Nm. This is still a lot, but the benefit is that the disk does not need to turn extremely fast, like would be desirable for the Tuatara. With gears or a worm drive, torques significantly below this 1023 Nm can be transformed into much higher torques. As an order-of-magnitude example, the following motor will be looked at.[17] The relevant parameters are the:

• Box volume (the volume of the smallest rectangular box one can place it in): 4.5 L
• Mass: 4.5 kg
• Power: 120 W
• Torque: 1.3 Nm
• Maximum speed: 850 RPM
• Input voltage: 230 V AC

The required torque factor is 1023/1.3=787. This is a lot of tooth for one gear, and it is intended to keep it compact. To that end, 2 the same worm drives might be used, each having a ratio of at least 28:1 (30 is a customary number of teeth, which is convenient as it thus goes about 15% over the required torque). These are available at industrial levels at diameters below 5 cm. If custom-made, they can probably be made to fit inside a volume of about 1 L. The total transmission then becomes 30*30=900. At this ratio, the disk would be made to turn at .944 RPM. That means that the disk will turn halfway in about 28 seconds. The total energy requirement for this turn will be 120*28=3400 Joules. A battery on board the submarine should be able to take in a sample and deposit it outside again at least three times in a row + some to spare to allow for repeated and thus more reliable sampling. Thus, the battery should be able to store at least 23800 (7 half turns) but ideally 34000 Joules of electric energy. 34000 Joules is not a lot. A typical AA rechargeable battery can easily store such energies. The problem is in the 230 V AC requirement, with which you are automatically looking at heftier battery packs, such as the following one.[18] In a custom design this can probably be made a lot smaller, especially if it needs only 1 or 2 output ports. The volume of this pack is 5.6 L and it has a mass of 5.4 kg. This number will be divided by 3 for our battery.

Communication

The communication chain will be divided into three parts; from Earth to surface receiver, from surface receiver to sub-surface receiver, and from this sub-surface receiver to the submarine.

From Earth to surface receiver

On Earth, NASA uses a system called DSN to communicate with spacecraft.[19] DSN stands for Deep Space Network, and it allows NASA to communicate with spacecraft as far as Opportunity 1, which is, by now, outside of our solar system. This means that a satellite, as intermediate station, around Europa is not absolutely necessary, but it may provide a faster way to get our data to Earth. Since Europa is tidally locked to Jupiter [20], and since its orbital period is about 3.5 earth days [21], a large part of the time, the lander will not be able to directly send data back to Earth. This is due to either Jupiter being in the way, or Europa itself is in the way when the lander is at the back of Europa, relative to Earth. If, for this mission, there was another satellite orbiting Europa or Jupiter, the lander would be able to send its data to this satellite with a much higher data rate. During the Mars Curiosity mission, NASA has an orbiter around Mars, which intermittently communicates with the Rover. The communications between Curiosity and this orbiter are about 150 times as fast as the communications would be between Curiosity and Earth directly. Since this orbiter has more power that it can focus on communications, the data will reach Earth much faster through this orbiter, than it would directly [22]. So, in conclusion, a satellite would increase the speed of communication, but it is not required.

Necessary bandwidth

The amount of bandwidth that is necessary to ensure that all collected data reaches Earth is of course difficult to determine beforehand. However, an estimation of the bandwidth that will be necessary can be made. It should be noted that the most significant hurdle that must be overcome when it comes to bandwidth, is to get collected data from the lander on Europa to NASA’s DSN on Earth. As for the acoustic communication between the submarine and the sub-surface station, Singh et al. [23] have shown a bitrate of 5000 bps at a range of up until 6000 meters, and a bitrate of 200 bps at a range of up to 11000 meters. Suzuki et al. [24] have managed to obtain a bitrate of 16 kbps at depths of more than 6500 meters. Since it would not make sense to send more data to the surface lander than the lander can send to the Earth, the bitrate that should be necessary from the lander to Earth should be the same bitrate. If this mission is to be played really safe, then the actual bitrate should be a little bit higher, to ensure that the lander gets to send all the data to Earth that it received from the submarine.

From surface receiver to sub-surface receiver

In this section different options of communication through this difficult medium will be discussed; wireless communication through ice, fiber-optic cable and electrical cable.

Wireless communication through ice

Ice is quite a good isolation material when it comes to wireless signals. Radio signals are very quickly absorbed by ice [25], and optical signals do not travel through ice very far either. Low frequency radio waves, however, do travel through ice better [26]. The question arises, however, whether these radio waves will survive through 10 km of ice, as that would be a very unusual situation here on Earth for researchers to test on. So it is assumed that wireless communication through the ice crust on Europa is for all intents and purposes impossible.

Fiber-optic cable

This a method of transmitting information from one place to another by sending pulses of light through an optical fiber. A fiber-optic cable was used in the ARTEMIS mission, where the navigation and communication under and through the ice in Antarctica was examined. The function of this fiber was to monitor ARTEMIS under water and allow the scientists to display the real time outputs of the sensors on a screen. There were high noise levels on some sensors. The fiber was 15 km long, but ARTEMIS was at its maximum 10 km from the base. Due to the ocean currents the fiber got damaged so it could not be re-used. [27][28] The ice of Europa is also believed to be moving around due to tectonic activity, tides and gravitational forces. Since the cable needs to last at least the whole 5 years of the mission, a fragile cable that is damaged only by the currents of the Antarctic sub-surface ocean is not an option.

Electrical cable

Quite the only method to communicate between the surface and sub-crust bases that remains is an electrical cable. To allow communication, it is important that sufficient signal can be transferred through the cables. To that end, the applied voltage should result in enough current to transfer the signal. This current can be determined using Ohm’s law: A=V/R Where V is the applied voltage and R the total electric resistance of the cable. R can be calculated using the following formula: R=l/a where ρ is the resistivity of the cable (a material property), l the length of the cable and a the cross-sectional area of the cable (π*r²). Substituting this: A=(πr^2/V)/(ρl) l is a given. It should be long enough that it can pass all the way through the Europan crust. Given some margin, l should be 11000 m. ρ is a material property, and there are limits to how conductive a material can get. Materials that enter the superconducting regime at 100 K are considered ‘high-temperature superconductors’. Now, temperatures on Europa’s surface do drop below 100 K at night, but can reach 135 K during the day, as well as rise as you dig deeper into the crust, all the way up until 273 K. The result being that for a superconductor to make sense, it needs to be a significantly better conductor than any other material at room temperature as well. For instance, as shown by Han [29], even though LaBCO makes the transition to superconductivity at 20 K (as opposed to many materials that require temperatures on the order of 1 K), its resistivity at room temperature is still 2 orders of magnitude larger than silver at 15.9 nΩm or copper at 16.8 nΩm. Hence it is proposed to use either of the two. The biggest difference between the two is in their densities. [30] Copper has a density of 8790 kg/m³, whereas silver has a density of 10500 kg/m³. The ratio between the resistivities is 1.057, between the densities 1.19. That means that even though a silver wire could yield the same resistance with a 5.7% slimmer (and thus less voluminous) wire, that wire will still be 19% heavier per unit volume and thus still be 13% heavier. That implies copper is the way to go.

For the coming calculations, it shall be assumed that copper has a constant resistivity of 16.8 nΩm. In practice this resistivity decreases slightly as the temperature decreases, but that means that the constancy assumption is a safe assumption. The resistance must preferably be as low as possible for as little mass as possible. To possibly be able to minimize both, an attempt will be made to relate the two. The mass of the wire is equal to m = ρ_mV = ρ_mal. Writing this as an expression for a and substituting in the expression for R gives: R = (ρ*ρ_ml²)/m This shows that there really is not an optimal mass for which R is lowest. Since direct reduction of the mass is probably best, the maximum R to allow signal transfer will be investigated, and the mass adjusted accordingly. To give a numerical example, a copper wire diameter of 1 mm will yield a 76 kg wire with a total resistance of 235 Ω. This is on the order of magnitude of an incandescent light bulb. m and R then scale inversely proportionally. Quadrupling the mass (double the diameter) will decrease the resistance 4-fold as well. The ultimate limit is determined by how high a R can be permitted, which is in turn determined by the available voltage and the required current.

From sub-surface receiver to submarine

The ocean on Earth contains a layer of water referred to as the SOFAR layer. [31] This is the layer of water where the speed of sound in the water is the lowest. This layer of water is also where sound travels the farthest in the ocean, tested up to about 5000 kilometers away.[32] Since the SOFAR channel in water is dependent on multiple factors of the water, it is not unthinkable that the ocean on Europa will also have such a layer. If this layer can be found in the ocean of Europa, it will enable the submarine to communicate with the subsurface station for a very long distance, with minimal loss of the sound signal through the water. There exist also formulas to calculate the propagation of sound in salt water, but because the salt content of the water on Europa is yet unknown, these formulas are almost useless. These formulas are taken into consideration in the discussion, but in conclusion, low frequency sound waves are the best option for this mission to enable very long range underwater communication.

Autonomy

This section will outline how much autonomy the robot must have. First of all, it must be calculated how long communication will cost between the robot and earth. The communication between earth and the surface-receiver on Europa’s surface can go via electromagnetic waves, which travel with the speed of light (299.8 *10⁶ m/s). Since the distance between earth and Europa is 628.3*10⁶ km, the signal will travel for 0.58 hour before it reaches Europa. The communication from the surface-receiver to the sub-crust base will go through an electrical cable, and since the estimated speed through an electric cable is 270*10⁶ m/s, the time it takes to travel 10 kilometers through the ice is negligible. For the communication from the sub-crust base to the submarine, acoustic waves will be used. The speed of sound waves is 1550 m/s, and the maximum distance the submarine will be apart from the sub-crust base is 4700 km, so the travel time of the signal through water can be as long as 0.84 hours. So with an one-way communication time of up to 1.42 hours, it will last up to 2,84 hours to receive a signal on earth and deliver a signal back to the robot on Europa.

Since this communication time is way too long for a fully human controlled robot, it should have some level of autonomy. For instance, when the submarine encounters a big object like a piece of ice, it should be able to go past it without ‘asking’ the controllers on Earth what to do. This will save lots of time. Therefore, the submarine must contain sensors connecting to self-deciding devices for small problems like this. Another point where the level of autonomy must be determined is in regard to the navigation. Since the submarine has quite a track to run, it should have a certain level of autonomy. The general path it needs to go should be programmed in the computer of the submarine, but the precise route the submarine has to take it should determine for itself.

Bigger decisions, like if the robot is on the right site to land and dig, and whether the sub-crust base is in the right position in the ice, should be a human call. Due to the high level of uncertainty in what the mission will find, the robot is bound to encounter other problems or unexpected things it was not programmed to deal with. In this case the robot should stay on the safe side and also contact its human controllers. Where to execute the experiments should be on human order too, so the human controllers on earth can see if the submarine is in the right place and environment to execute these experiments.

However, it should be possible to change the pre-programmed track at any time, as some unexpected events may occur that require a change of track or shortening or elongation of the mission. In this case, commands will have to be sent to the robot, from Earth, to override its scheduled tasks. If these commands are comparable to those given to computers through, say, Command Prompt or Terminal, these commands are up to several tens of characters long. These characters are then encoded in Unicode (UTF-8), which encodes the first 127 characters (the only ones that are necessary) in 7 bits.[33] Depending on the length of the command, a multiple of 7 bits is necessary per command, or 7n, where n is the amount of characters that the command consists of. Although the download of these packets at the submarine side of the connection to Earth occupies bandwidth as well, the main connection limited by the bandwidth is the sending of data from the submarine to the sub-crust base. The connection the other way around is much less used and will thus have ample space for short unicode codes.

Extraterrestrial life

According to the precautionary principle, if human activities may lead to morally unacceptable harm that is scientifically plausible but uncertain, one should take action to avoid or diminish that harm.[34] Since there is a lot of uncertainty about the possible life on Europa, it is very important to explicate the moral value of extraterrestrial life, all forms of life the robot could encounter and how it is prevented to damage the life or environment on Europa.

To begin, the moral value of extraterrestrial life. The following dissertation outlines why all life, terrestrial or not, should be considered to have some intrinsic value. This view is adopted, acknowledging that at the same time extraterrestrial life has extrinsic value as well, as it can serve to further scientific research as argued in the following article. [35] Furthermore, one should note that, if something does not have intrinsic value, this does not mean it should not be preserved. If something has value to something with moral value, it acquires value. So it also goes for the Europan environment, which is important for the life present on it, as well as for our own research purposes. So what could be the different forms of life that the robot might encounter during the mission and how should each form of life be dealt with?

First of all, sentient versus non-sentient life. If life is found at all, it is far more likely that it will be microbes or other non-intelligent alien life than sentient otherworldly beings as argued here.[36] As stated in previous paragraph, all life should be considered to possess intrinsic value, which means that the potential sentient and non-sentient life should be treated the same way. In the previous section experiments, one can read the experiments that are planned to be examined on Europa. None of the experiments will harm or disturb the potential life directly. But the life can be harmed indirectly too, which will be explained later in this section.

Second of all, carbon-based life versus non carbon based life. On earth, all known living things are based on the element carbon. But scientists belief that there could be an alternative chemical basis for life, for example silicon-based life, described in the following article.[37] There could be creatures in unimaginable forms and capabilities on Europa, but since it is also “life”, these potential creatures will be treated the same as carbon-based life, with the assumption that they have some sort of intrinsic value. Another thing that needs to be excluded, is the possibility of contamination and thereby affecting Europa’s environment or ecosystem. This is the indirect harm of life on Europa as addressed before. Contamination can be done in different ways; via human bacteria or viruses that end up on the robot during the construction of it, wear or rust of the metals in the robot or a radioactive source that poisons the environment. The first possible way of contamination, human bacteria, seems to be no problem at all. According to the following source, bacteria die slowly when they are exposed to freezing temperatures.[38] And since the robot is traveling through the space for about 6 years in -270 degrees of Celsius, it can be assumed that all possible human bacteria are dead when the robot arrives on Europa.

Viruses are a higher risk for contamination. There are multiple researches done to the surviving of viruses in spatially conditions, and some experiments proved that viruses can actually survive in these conditions for a long time. The longest time a virus was exposed to (and survived) the space environment was 2 years. Since there is no data of the decline of this specific virus, it is not possible to make a prediction if this virus will survive the 6 travel years to Europa. And thereby it can not be excluded that some viruses might be transferred from earth to Europa during the mission. The only thing that can be done, is to make sure that the robot goes into space as virus-free as possible. As can be read in the following article, there is also a possibility that the robot could catch a virus on its way to Europa.[39] But to date, almost no research has looked into the possibility of viruses “living” in space. So until this remains unknown, it is unknown if this risk needs to be taken into account.[40]

For the last two ways of contamination, wear or rust of the metals in the robot or a radioactive source that poisons the environment, the choice for materials and energy source should take these dangers into account. So the materials should be the best non-wearing and non-rusting materials available. And an energy source that does not have radioactive leakage must be used, so the environment of Europa will not be in danger. This will be taken into account in the section on power sources.

How long does the mission need to last?

Europa could possibly be regarded as being spherically symmetric, were it not for Jupiter’s strong gravitational influence on her. Many processes are probably highly influenced by Jupiter, either positively or negatively. Since it is uncertain which processes can sprout life and/or how those processes are influenced by external gravity, it can be interesting to search for life both on the Jovian and the Anti-Jovian side of Europa.

Figure 4: 8 interesting points on Europa

Figure 4 to the right is the same image as Figure 4. The surface points indicate the surface of the ocean, below the crust, and are noted as Jovian (J), Anti-Jovian (A), North (N) and South (S). The seafloor points have the same letters, shifted 1 forward. To search the Jovian and Anti-Jovian poles, the lines AB and JK make the most sense. Have the submarine sail across these lines and take a sample every so often. To travel between these lines, the semicircle BOK or BTK seems most logical because the radius of these circles is the smallest possible. The total route ABOKJ is 4700 km. However, that does require that the submarine can remain at seafloor levels of pressure for extended periods of time (on the order of years). To reduce the time at full depth, the route ABANJK can be taken. This one, however, is 5320 km. The observant reader may have noticed that 5320 km is A LOT. A car driving on the Dutch highways will have to drive from Groningen to Maastricht and back almost 8 times. This will take roughly 60 hours. Bathyscaphe Trieste (the first submersible to reach the bottom of the Challenger Deep) submerged and resurfaced with an average speed of 0.9 m/s, with which it would complete the journey in 68 days, ignoring the fact that Trieste could only go up and down. The Trieste submersible had a mass of 14 metric tons. This is obviously much too big. However, it was made to harbor 2 people and protect them from the immense pressure, which is not needed. The inside pressure needs only be small enough that the equipment can still function. On the other hand, our submersible needs to withstand double the pressure that Trieste did. Furthermore, Trieste used gravity to sink and rise, which is much lower on Europa. All in all, the maximum diving speed of the submersible may cap out well below 1/10 of Trieste’s speed. Furthermore, the lateral speed of the submersible is probably even lower, due to complicated driving systems. Since the exact velocity of the submarine is difficult to estimate precisely at this point, this 0.05 m/s is used as the actual top speed. At this velocity, the long route takes 3.37 years, and the short route ‘only’ 2.98 years. Only going back and forth between the ice and the seafloor takes 70 days. This is a total distance of 300 km. Please remember that this is calculated in a straight line, completely ignoring the fact that the route straight down may not be possible due to obstructions, or desirable due to interesting finds along the way. All this also disregards the dig down to the sea. Valkyrie achieved a velocity of 0.9 m/hr. This means it would take 46 days to dig through the crust. Combine this with a startup time, communications check, and pre-disembarking measurements, and the total time before setting off could easily be 60 days. Considering the interest in both the Jovian and anti-Jovian poles of Europa, the ATOKJ is likely most useful. To allow for deviations, an estimated 41% length will be added to the route; this will allow a deviation off the route of 45 degrees at all times. At an average speed of 0.05 m/s, this will take an estimated 1538 days, or 4.21 years. Add the 60 days to get 4.4 years. Plus some room for error, the mission will last between 4.5 and 5 years.

From Earth to Europa

This research focuses on the landing on Europa, digging through the icy layer and sending a submarine into the sub-surface ocean. As the digger and submarine are the most novel ideas of the research, the main focus will be on those components rather than on the surface lander. Similarly, the trip from earth to Europa will not be described in much detail, because previous spacecraft missions have shown the possibility to travel this far. There is one important constraint to take into account; the maximum payload a rocket can send to Europa. The most powerful operational rocket to date is the Falcon Heavy rocket, which can take twice as much payload into space as any other rocket.[41] It is unknown exactly how much payload the Falcon Heavy rocket can take to Europa, but it can take a maximum payload of 63800 kg into a low earth orbit, 16800 kg to Mars and 3500 kg to Pluto. Since the maximum payload seems to decrease exponentially as the distance increases, the assumption is made that the maximum payload that can be delivered to Europa is also 3500 kg. The true value will probably be a bit higher, but 3500 kg is a safe estimation. With the help of the rocket, the spacecraft makes its way into space. With a varying travel speed it will cost the spacecraft about six years to reach Europa. During this travel time it has a very little, negligible chance of bumping into some meteorite or something else in space. [42]

Landing and surviving on the surface

Where to land?

This research focuses on the landing on Europa, digging through the icy layer and sending a submarine into the sub-surface ocean. As the digger and submarine are the most novel ideas of the research, the main focus will be on those components rather than on the surface lander. Similarly, the trip from earth to Europa will not be described in much detail, because previous spacecraft missions have shown the possibility to travel this far. There is one important constraint to take into account; the maximum payload a rocket can send to Europa. The most powerful operational rocket to date is the Falcon Heavy rocket, which can take twice as much payload into space as any other rocket.[43] It is unknown exactly how much payload the Falcon Heavy rocket can take to Europa, but it can take a maximum payload of 63800 kg into a low earth orbit, 16800 kg to Mars and 3500 kg to Pluto. Since the maximum payload seems to decrease exponentially as the distance increases, the assumption is made that the maximum payload that can be delivered to Europa is also 3500 kg. The true value will probably be a bit higher, but 3500 kg is a safe estimation. With the help of the rocket, the spacecraft makes its way into space. With a varying travel speed it will cost the spacecraft about six years to reach Europa. During this travel time it has a very little, negligible chance of bumping into some meteorite or something else in space. [44]

Through the ice - from surface to sub-surface ocean

Power

The first question to ask is where to put a power source and what power source to use. Valkyrie, for instance, used laser power to dig through the ice. The laser light was produced at the surface and travelled all the way down the optical fibre to there melt the ice in front of the digger. However, first of all this is probably only possible with an optical fibre, not with an electrical cable. The only option to power the digger from the surface that remains is to send all power through the electrical cable. However, this will require a conversion of whatever power is used to electrical power, which always comes with a percentage in loss. Furthermore, the electrical cable will result in quite some losses as well. Therefore it is proposed to put a power source in the digger. It is not feasible to rely on finding combustible chemicals on Europa as part of the mission is to determine what chemicals are present. The digger will also for the most part be beneath kilometres of ice so solar power is not an option either. That leaves nuclear power. One benefit is that a large nuclear power source is heavy. Placing this in the front of the digger will weigh its front down, making sure that it digs straight, in much the same way that the relatively heavy head of a badminton shuttle helps orient it. Furthermore, placing such a large power source in the digger allows one to simply tap off a very small percentage of the power (for instance 1%) using a thermocouple. If the power source produces 1 MW of total power, the thermocouple will generate 10 kW of electrical power, at a meagre loss of digging power (which is the remainder thermal power). Thus, it is proposed to place a nuclear power source in front of the digger, rather than on the surface. The details of this source will be outlined in the following paragraphs.

Model for digging through the ice

Figure 5a: Fuel source of 1 kW in the front

Figure 5d: Fuel source of 100 kW in the front

Figure 5b: Fuel source of 4 kW in the front

Figure 5e: Fuel source in the front with a hollow cylinder along the length

Figure 5c: Fuel source of 10 kW in the front

Figure 5f: Fuel source divided into two rings of 4 kW

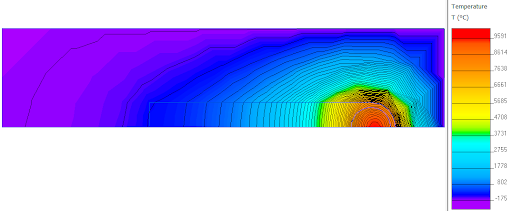
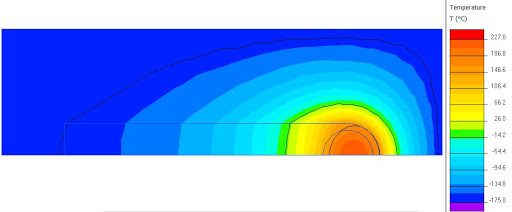
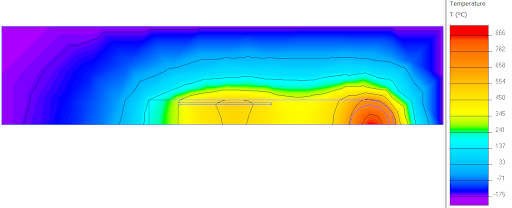
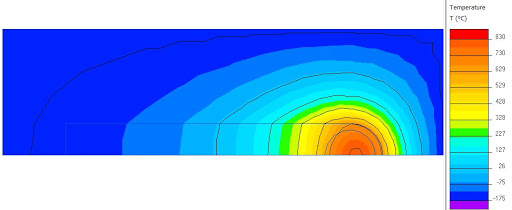
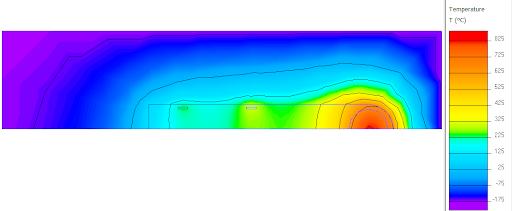
In this case it is important to consider the balance between influx and outflux of energy. The digger needs to heat the ice in front of it enough to melt and possibly vaporise it, and the ice around it to allow the water to pass it by. At the same time, the surrounding ice tries to take away energy, preventing the water from freezing. Because QuickField cannot model phase changes, the phase changes will be approximated by a temperature difference. The latent heat of fusion of ice is 334 kJ/kg.[45] At 0 degrees Celsius, ice has a specific heat of 2.050 kJ/kg K.[46] Dividing l/C_v gives ΔT=334/2.050=163 K.

That means that in the model, temperatures between 0 and 163 degrees Celsius correspond to a phase change between ice and water. Below 0 degrees is pure ice of temperature T, and above 163 degrees is pure water of temperature T-163. This is by no means scientifically sound, but does serve to give an order-of-magnitude indication of how the temperature works.

The pictures 5 a, b and c display a source in the front of the digger, with a total output of (from top to bottom) 1, 4 and 10 kW respectively. Furthermore, the isotherms in the picture are spaced by 163 degrees Celsius, starting at -163 degrees. That means that the outermost isotherm indicates still far below freezing temperatures, but the 2nd and 3rd outermost isotherms indicate the lower and upper edges of the freezing regime. This shows that at 1 kW, the digger is unable to melt any of the surrounding ice. At 4 kW it is visible that a small layer just in front of the digger will melt completely. However, as the digger would progress and this water would flow to the back of the digger, it will quickly return to the half-molten regime. It is only at 10 kW that the digger forms a significant layer of fully molten water around it, with a thickness of between 10 and 20 cm. However, not even halfway down the length of the digger, all this water will refreeze. As shown in picture 5d, to warm the entire digger 100 kW is required. This is obviously a lot, and based on the model a large portion of the equipment would reach absurd temperatures. Furthermore, in the model, convective processes are neglected so the actual necessary energy could even be a factor of order 1 larger or smaller. To overcome this, a very small, long heat source along the length of the digger is proposed. In this case, the same water layer can be established, but with only 20% the total power: 10 kW for both sources. Bear in mind that this power source is a hollow cylinder. The last configuration is one in which the cylinder has been replaced by two rings that produce 4 kW each. This requires less power but gives a similar temperature profile.

Furthermore, from the power output of the source, the maximum speed of the digger can be determined. This is based on the amount of ice in front of the digger that it can melt per second. The energy H required per kilogram of ice to melt it is equal to: H = l_f+C_v*ΔT, where l_f is the latent heat of fusion, the energy required to induce a phase change, C_v the heat capacity and ΔT the temperature difference to be established. for ice, l_f=334kJ/kg, C_v=2.05kJ/kg/K and in the case of Europa ΔT=50 -- 200 = 250K at least. The lower temperature boundary is expected to be the lowest temperature that will be encountered on Europa, whereas the upper temperature boundary is there to ensure that the water stays molten for a while. This temperature difference will likely decrease as the digger gets deeper into the crust, because the ice warms up slowly. However, in the worst case this will allow the digger to go somewhat faster. The total energy then becomes H=334+2.05*250=846.5kJ/kg. The energy per unit volume can be determined from the density of ice: 920 kg/m³, resulting in 778780 kJ/m³. The digger’s speed v can be calculated by dividing the total power used to melt the ice directly in front of it P by the energy required per unit volume H and the total frontal area of the digger A. The latter is equal to π*r², where r is the radius of the digger's cylindrical part. v = p/(H*π*r²) For instance, at 10 kW and a radius of 25 cm, the velocity of the digger becomes 65 μm/s. At this velocity, the digger would take about 4.8 years to dig 10 km. At 1 MW effective power, this is reduced to a much more manageable 17.7 days. Thus, even though a 10 kW power source would be sufficient to be able to dig at all, it is proposed to use a 1 MW power source.

Constructing the power source

For power sources up to tens of kilowatts, radioisotope thermonuclear generators (RTGs) are used. The determination was based largely on research done by M. Ragheb. [47] If not mentioned otherwise, radioisotope data in the remainder of this section is derived from this document. There are several consideration to be made when selecting a power source for an RTG:

• Sufficient (power) density
• Sufficient duration
• Right type of radiation
• Non-critical

(Power) density

In particular in space missions, the power density of the source is important. For instance, it is possible to get a block of Am²⁴¹O₂ that produces 10 kW, but at a power density of .097 thermal Watts per gram, this block would weigh 103 kg. As a comparison, Am²⁴⁴₂O₂ has a power density of 2.01 Wh/g, resulting in 10 kW of power at only 4.98 kg. Furthermore, the material's density itself matters as well. To illustrate: Cs¹³⁷Cl has a power density similar to Am²⁴¹O₂, but not 1/3 the density. As a result, a block of Cesium Chloride must be 3 times as large to produce the same power as a block of Americium Dioxide, whereas both blocks have about the same mass. Thus, the perfect power source should have a large enough power density to decrease its mass, as well as a high enough mass density to decrease its volume. The mass of the power source is equal to the desired power divided by the power density. This mass should be divided by the mass density to find the volume of the power source.

Duration

One disadvantage of RTGs is that due to the decay of the radioactive source, the power of the source slowly decreases over time. An isotope with a sufficient half-life should thus be chosen accordingly. Consider 3 isotopes:

• Uranium-235, with a half-life of 8.9*10⁸ years[48]
• Plutonium-238, with a half-life of 87.74 years
• Cerium-144, half-life of 284.4 days.

The activity of isotopes is proportional to the number of isotopes. In particular: A(t) = A(0)*2^(-t/t_(1/2)) with A(0) the initial activity (to which power production is proportional) and t_(1/2) the half-life of the radioactive source. Filling in the half-lives for t = 7 years, a rough estimate of the travel time to Europa, results in activities at the time of arrival of 1, 0.946 and 0.00198 times the initial activity for U-235, Pu-238 and Ce-144 respectively. This goes to show that an isotope like Cesium-144 would reduce in power by 99.8% over the course of the journey to Europa. To achieve a power of 10 kW upon arrival, the initial power source must then be 5 MW. This power requires a 200 kg Ce source. Uranium and Plutonium, on the other hand, retain most of their activity over the course of the mission. However, this would imply that Uranium would be a better source than Plutonium. This is not the case. Even though the activity of U decreases more slowly than Pu, the activity of Pu is higher than U. This can be understood by realising that U has a longer half-life ‘’because’’ it decays slowly. If it decayed faster, all the atoms would deplete in a much shorter timespan. There is a general inverse correlation between activity and half-life. As a result, the half-life of the perfect isotope is too high nor too low, preferably about an order of magnitude greater than the duration of the mission. The power density upon leaving earth can be calculated as follows: P(t) = P(0)*2^(-t/t_(1/2)).

Type of radiation

Now the type of radioactive decay that the perfect radioactive source undergoes shall be considered. To avoid radioactive contamination of environments or the destruction of on-board equipment, it is important to shield the radioactive source. At the same time, due to weight considerations, it is also important that said shielding is not too thick. To achieve the latter, the right type of radiation has to be chosen. Of the four main types of decay - alpha, beta, gamma and neutron - alpha is by far the most easy to mitigate, with air half-value layers of the order of cms. Hence, it also makes sense to choose a radioactive source that decays via alpha radiation. Examples are Plutonium-238 and Curium-244.

Non-critical

Lastly it is important that the mass of the radioactive isotope does not exceed its critical mass. If a hunk of the isotope exceeds the critical mass, the decay of atoms in the isotope will accelerate the decay of other atoms in the isotope, causing a runaway chain reaction like the ones that occurred in Tsjernobyl and Fukushima, like the ones that are induced in nuclear bombs. If the mass is kept small enough, or the geometry is chosen efficiently, the atoms do not interact with each other as much and the chain reaction will remain under control. These comparisons hopefully suffice to illustrate why a runaway chain reaction is undesirable. The considerations to be made are as follows:

• ’’High critical mass of the isotope’’: This allows for a larger amount of isotope to be taken along on the mission.
• ’’Geometry’’: As in a spherical configuration the atoms are most densely packed together, the critical mass is lower in a sphere.[49]

This in particular limits the size of RTGs. If the power is to increase, the fuel mass has to increase too. To avoid exceeding the critical mass, this fuel then has to be subdivided in smaller portions which all require independent shielding. This in turn causes the amount of shielding required to scale faster than linearly with the mass. As a result, too big an RTG will simply be too heavy.

The best power source

Below is presented an overview of the most promising radioactive sources, with from left to right their half-life, power density, mass density, critical mass, P0, mass, volume, attenuation layer. To determine what source is ‘most promising’, the right principal type of radiation and the right half life of some isotopes were picked from the article on RTG source selection mentioned in the beginning of this section. Most information was gathered from that article as well. The isotopes chosen were Curium-244[50], Plutonium-238[51] and Americium-241[52]. It should be noted that the following discussion concerns itself with designing a 10 kW RTG. This is thus mainly for the purpose of increasing the power source in the submarine, or decreasing that in the digger. To determine the attenuation layer, the lead layer thickness required to reduce the gamma dose (alpha does not transmit through lead at all) at 1 m to .1 mSv. Rimshaw and Ketchen showed that the shield required to meet precisely that condition for Curium with lead has a thickness of 11 cm.[53] For other sources this information appears impossible to find. Thus, to get an order of magnitude estimation of the other materials, the ratio between this value and the values listed in the article on RTG power sources will be used. Since the attenuation thickness for Cm-244 as listed by Ragheb is 5.11 cm, this ratio is 2.155. All attenuation layers listed by Ragheb will be multiplied by 2.155, results are in the table.

	t(1/2) (a)	ρ (kg/L)	ρw (W/kg)	mc (kg)	P(0) (W)	m (kg)	V (L)	r (dm)	d (dm)	ml (kg)
Cm	18.11	13.51	2840	20.07	13072	4.6	0.341	.433	1.1	167.4
Pu	87.74	19.33	560	8.2	10568	18.9	0.976	.615	0.0547	3.224
Am	432.0	13.66	110	56.4	10113	91.93	6.73	1.17	.3832	102.1

The two most important conclusions to be drawn from the table are the following: Cm, at the required mass, weighs less than a quarter of its critical mass. Pu and Am have approximately 2 times the mass. So, Cm can be used as a solid sphere, whereas for Pu and Am, a different geometry would have to be chosen, or multiple smaller spheres have to be used. Furthermore, disregarding the critical masses of the isotopes, Cm would require a sphere with a radius of 15.3 cm, weighing 172 kg; Pu a sphere of radius 6.7 cm, weighing 22.1 kg; and Am radius 15.5 cm, weighing 194 kg. Considering the critical versus required mass of Americium, it can be quickly dismissed as a useful source. Thus, to choose between either Cm or Pu, it will be attempted to make different suggestions for the geometry of the Plutonium, and determine whether the different geometries still result in lower masses than Curium. The first is to split the single plutonium core into 3 separate cores. The mass of these will be the same, but each will now have a radius of 4.27 cm. To coat these in a layer of .547 cm of lead, requires 1.6 kg each, or a total of 4.8 kg, bringing the total mass up to 23.7 kg. However, this configuration does not lend itself for a very homogeneous temperature distribution. For that, a hollow hemisphere with a thickness of half the critical radius (rc=4.66 cm) will be used. To obtain the required mass of 18.9 kg, this sphere should have an inner radius of 6.97 cm. To coat this hemisphere with the required layer on both in- and outside (the outside for protection of the environment, and the inside for protection against itself), requires 10.0 kg of lead, for a total of 28.9 kg. The last suggested configuration is a torus with a square cross section with a diagonal cross section equal to the critical radius as listed here (4.04 cm).[54] The major radius of this torus is then 9.52 cm. To cover this torus in lead, requires 6.8 kg of lead, bringing the total mass to 25.7 kg. Each of these configurations has its own benefits and disbenefits. The 3 spheres probably do not create a very homogeneous temperature distribution, which may cause the digger to lose efficiency when digging, or have trouble going straight. The hollow sphere is the heaviest of the 3 configurations, and the torus fails to heat the front of the digger, where the heat is needed most. The best solution can be found in a combination. The idea is to create a sphere of plutonium with a torus around it. The sphere and torus are separated by a double layer of lead, then the radii are varied to get the exact right mass of plutonium. The cross section of the configuration is shown in the figure below:

Calculations give a radius of the sphere of 4.47 cm, still below the critical radius, and a major radius of the torus of 7.04 cm. The total mass of shielding is 6.4 kg, resulting in a total of 25.3 kg. This is still a bit above the mass of the spheres, but a little below the mass of the single torus. The configuration is radially symmetric, and due to the smaller torus radius can be placed higher up in the digger.

Figure 6: Construction of the plutonium sphere and torus

Furthermore, if deemed necessary for sufficient heat transfer, the sphere can be pushed up a little further than the torus as well. This way the Plutonium-238 does not reach critical masses, and the total mass is still well below the mass of the total Curium-244 source.

Now, as mentioned before, this is not the power source suggested for the final design. This is an outline of the considerations to be made when working out the details of the mission shows that either the submarine needs more power or the digger can do with less power. To conclude that, the best 10 kW-range power source was found to be Plutonium-238, due to its high alpha ray energy density. The configuration of source material best suited for these purposes is the sphere-torus combination. However, this examination showed that more power requires disproportionately more shielding. It was shown that the 10 kW power source would have to be broken down into at least 3 separate spherical power sources. A 1 MW power source would have an initial mass of 1890 kg, which is equivalent to 230.5 critical masses. To break this down into spheres would result in spheres of 423 cm³ each, with a radius of 4.66 cm. The shielding required for this has a mass of 3.89 kg for every sphere. Times 231 spheres results in 900 kg of shielding. That means that only the power source of the digger would weigh a total of 2790 kg, which is 80% of the total mass that can be brought to Europa. Needless to say that this will not be a viable option for the mission. To solve this problem, NASA is designing power systems for space missions that are able to provide this 1 MW (and more) for extended periods of time. [55] The selection of these systems, however, is considered outside the scope of this project. This is in part due to the complexity and in part due to the fact that these systems are still being optimised. The paper cited above discussed reactors that produce up to 15 MW of power with a mass of 2 metric tons. Scaling this down most likely won’t go linearly, but it will be assumed that the power scales with the square of the mass. Thus decreasing the power by a factor of 15 will reduce the mass to about .5 tons. Assuming that the reactor has an average density half the density of plutonium, the reactor will have a volume of 60.6 L. However, should it at some point be decided that the power source in the submarine should be increased, or the power source in the submarine can be decreased, the above gives a suggestion on how to do this.

Sub-crust base

Once the digger has dug all the way through the ice, it anchors itself into the ice with a system similar to the one in in this paper.[56] Once it has fully anchored itself, it opens the compartment that the submarine is contained in. The part of the digger that remains anchored to the ice, becomes the sub-crust base. This base then communicates through acoustic waves with the submarine, while being tethered to the surface lander through an electrical cable.

Where to place the sub-crust base

To allow for a sub-crust (SC) base that maintains wired contact with the surface base, the SC base must maintain a fixed position with the cable locked inside the ice. Otherwise the connecting cable is highly likely to break due to convective stresses. Another solution would be to make a (partially) stronger cable that can withstand these stresses, but this will increase the carry load and if the strong cable is somehow detached from the ice, the cable will break anyway. However, the submarine does need to be able to detach from the SC base and sail away. Most conveniently, the SC base would be half still lodged in the ice, and half sticking out into the sea below the ice. Considering this, a problem arises. The viscosity of water increases with increasing pressure [57], as well as with increasing salinity: The viscosity of seawater is up to 8% higher than that of pure water. This means that the ocean just below the Europan crust may have a significantly higher viscosity than pure water. Furthermore, erosion due to convective processes in the ocean may result in a slushy water texture just below the Europan crust. If this is indeed the case, it adds a different requirement, namely for the submarine to be able to navigate and communicate through slushy or viscous water. There is no gradient transition between ice and water in a frozen lake. This means that once you are through the ice, there is only more water, not some substance that is chemically in between ice and water. Due to the scale and tectonic activity of the ice crust on Europa, this might be entirely different, but the Earthly example would imply no gradient. Furthermore, as of yet, the most probable explanations for ridge formation would not work with a viscous or slushy subsurface ocean, or a crust up to 10 km thick. [58] It is thus unlikely that the mission will encounter problematically viscous water.

Underwater Movement Submarine

Movement under water

To be able to manoeuver under water, the submarine needs to have a propulsion system. The simplest propulsion system that would (probably) work, is one (or more) propellers, like the ones that submarines have. Underwater drones are already available to the public, and most of them seem to feature this style of propulsion. Another alternative propulsion method may be one that is designed by means of biomimicry. One example of such a project can be seen to the right. In this image, the drone mimics the movements of a squid to propel itself forth.NOA Marine Besides this design, there have been multiple other designs that rely on biomimicry, for example mimicking a manta ray.

Figure 7: prototype of biomimetic propulsion system

As for the design of the propulsion system or our submarine, it is comprised of four turbines with propellers, oriented downwards, similar to how many quadcopter drones are built. These turbines can all four be tilted 90 degrees clockwise and anticlockwise, on the axis that they are connected to the submarine body with. It goes without saying that these turbines can be activated independently at will. This way, side-to-side, up-and-down, and forward-and-back movement can all be achieved.

Underwater thrusters for maneuverability

In order for the submarine to maintain its maneuverability under water, regular thrusters with propellers are incapable of withstanding the extreme conditions that the submarine will very likely encounter at certain depths in the ocean of Europa. Instead, underwater thrusters are required, which have a different design from conventional thrusters. (cannot find a more specific difference than that underwater thrusters have the ability to work under heavy water pressure, sometimes up to full ocean depth, also cannot find a specific difference in design).

Movement in salt water

Since our submarine will be using propellers driven by electric motors for movement underwater, these motors will have to be protected well from the saline water. Not only does the salt water corrode any electric circuitry, it also conducts electricity well. This conductivity may very well to lead a short circuit, definitely damaging (part of) the submarine. The corrosion will also definitely not be beneficial to the mission, as it may also cause the submarine to malfunction. Therefore, the electric motors (or any circuitry, for that matter) should be very well guarded from the salt water. As for the magnets inside the electric motors, there should not be any problem using them in the salt water of Europa.

Navigation under water

Figure 8: density of the water in Europa’s ocean

To allow navigation up and down, it is most convenient if the submarine will stay afloat at rest. For this, it should have a density equal to that of water. But water, although it has a low compressibility, is not entirely incompressible. As Fine and Millero showed, the specific volume of water decreases (and thus the density increases) as the external pressure increases. [59] Due to this fluctuation in density, the buoyant force on the submarine will increase as the submarine goes deeper down (where the pressure is higher). Hence, if the submarine floats at crust level, it will be pushed up. From the data of Fine and Millero, a pressure dependency of the density of water can be derived: ρ =1004.79*exp(3.20629*10^-5p) With ρ the density in kg/m³ and p the pressure in bar. (The observant reader may note that at p=0 Pa, the density of water is 1004.79 kg/m³, rather than 1000. That is due to the fact that an increase in temperature accompanying the increase in depth was taken into account. The first data point is at 16 MPa, 0 degrees Celsius. Supposing you could then decrease the pressure on the water, the function assumes that you are also decreasing the temperature, which results in a density increase. However, as the pressure in Europa’s ocean will not decrease below 16 MPa, the function works just fine in the relevant regime.) The figure below shows ρ(p(r)); the density of the water in Europa’s ocean between the seafloor and the sub-crust surface. Ideally, the submarine itself has a density a little over 1100 kg/m³, making it sink. However, if the submarine carries a kind of balloon filled with a material X with the following properties: X has a lower density than water X has a higher compressibility than water. What ideally happens is that the balloons are directly attached to the submarine. Their total mass is m. The volume of the submarine is V0, the balloon’s is V(p), so that the density of the combination is ρ=m/(V0+V(p)). V(p) is inversely proportional to the pressure, so as the pressure increases, V(p) decreases, so the density goes up. If this decrease in V(p) can be made to occur in such a way that the average density of the combination is at all times equal to the density of the water it is floating in. Let’s illustrate this with an example. Suppose the submarine has a mass of 750 kg, and a volume of .5 m³. The balloon has a mass of 50 kg. The density of the combination is then: ρ(p)=800/(0.5+V(p))=1004.79*exp(3.20629*10^-5p), Where the last equality follows from the density of water. Rewriting this equation results in a formula for V(p): V(p)=0.7962*exp(-3.20629*10^-5p)-0.5 Filling in a few particular values gives V(1)=.2962 m³, V(160)=.2921 m³, V(3000)=.22317 m³. The density of X as function of pressure then becomes: (p)=50/(0.7962*exp(-3.20629*10^-5p)-0.5) An idea for materials with the required densities can be acquired on the following site.[60] The accurate value of the density of suitable materials can then be found in literature. [61]

As it requires very accurate knowledge of the dimensions of the system, this section only serves to illustrate the concept. The actual numerics will be presented later on.

Is it possible to go to the seafloor?

There are 2 main theories for the origin of life on Earth: The prebiotic soup theory and the pioneer organism theory. [62] The latter is based in a hot, volcanic and sulfur-rich world. Considering the abundance of sulfur on Europa’s surface and the likelihood of Europa being heated by its own core, a pioneer organism process could take place on Europa. Hence, to find life it is worthwhile to investigate the bottom of the ocean, where said heating takes place. [63] The question that immediately arises is whether this is possible. Europa’s oceans are estimated to be 150 km deep. On earth, this would result in a hydrostatic pressure of roughly ρgh=1000*9.81*150,000=1.4715 GPa=14,516 atm. A quick estimation of the pressure in the sea demonstrates that pressure increases roughly linearly with increasing depth as you travel from the surface to the bottom of the sea. Just below the crust, the pressure is between 104 and 161 atmospheres, depending on whether you are on the sub- or anti-Jovian side respectively. This is equivalent to a depth of 1 and 1.6 km in Earth’s oceans. At the bottom of the sea, the pressure ranges from 1961 to 2961 atm, depending on the side, ranging between 20.2 and 30.6 km in depth. In principle, building a vessel capable of withstanding these pressures is possible. However, it should be noted that such vessels are generally extremely heavy and can only sink and rise once before needing to be reweighted for another dive and more importantly, they generally can not move laterally.[64] Taking in and expelling water at these pressures for propulsion is very difficult, as in order to be able to expel water, a force greater than pressure x area needs to be exerted outward. For an example, at 0.2 GPa, the force exerted on 1 cm² is 20,000 N. Furthermore, holes in the hull compromise the structural integrity, making it more prone to collapse and thus requiring a stronger (and thus presumably heavier) hull. Propellers with an internal motor will be prone to the same problem, which isn’t overcome by moving the motor outside the vessel, as then the motor needs to be a high-pressure vessel itself. A way to overcome this is by introducing driving the propeller with a magnetic field that reaches through the hull of the vessel. Furthermore, the required maximum pressure can be reduced significantly if deep-sea dives are only done on the sub-Jovian side, where the pressure may be more than 30% smaller than on the anti-Jovian side.

Design

Design of the surface lander

In light of novelty of research, the decision was made to focus on the digger and the submarine of our mission. The lander that is envisioned consists of three different parts that, at first, land on the planet as one unit. The first part, which contains the other two parts within itself, is the station. This station is envisioned as a quadruped, stationary structure. The four legs function as stabilization, making sure the station remains upright on the surface. Once the station has landed on the surface of Europa, it will remain stationary. This means that there is no use for any locomotion utilities on the lander. The envisioned design of the station reminds one a little of the Apollo Lunar Module. However, since there does not have to be a enclosure to harbor any astronauts, this station can be made smaller. This station is furthermore equipped with a few tools to perform some experiments on the surface, namely a seismometer and (a) camera(s). Contained within the center of this station is the digger, which makes its way through the solid ice. The station itself will remain on the surface, whilst physically remaining connected to the digger. This physical connection consists of a wire (fiberglass or electrical) that runs between the digger and the lander. This physical link was chosen because of the ice on Europa. This ice will most likely freeze shut any tunnel that the digger makes, which makes a wireless connection through the ice extremely unusable. This cable is, furthermore, unwound from the digger, not from the lander. This ensures that the least amount of stress possible is put on this cable. When the digger reaches the underside of the ice and is about to enter the water, the digger locks itself into the bottom of the ice. Inside this digger is a compartment that houses a submarine. This compartment will open to let the submarine into the water. The digger itself will remain stationary in the ice and communicate through sound waves with the submarine. The submarine, then, will start exploring the subsurface ocean. The submarine is outfitted with four turbines that can all be actuated along the axis that they are connected to the submarine along. The submarine, furthermore, carries (a) balloon(s), that inflate and deflate, depending on the depth that the submarine is at. During its exploration, this submarine will take pictures and perform spectrometric experiments and gas emission experiments.

Parts lists

Surface base

• Fixation stuff
• Power source
• Communications
  • Emitter
  • Receiver
• Research equipment
  • Seismometer
  • Spectrometer
  • Camera

Digger

Part	Subpart	Volume	Weight
Power source	Shielding	60600 cm3	500 kg
	Thermocouple
Accelerometer		In computer	Negligible
Fixation stuff		L = 5 cm	22.95 kg
Computer		100 cm 3	.3 kg
Communications	Emitter	250 cm3	.2 kg
	Receiver
	Cable	10,000 cm3	76 kg
Submarine		141,000 cm3	202.7 kg
	Fillers	163,500 cm3	147.1 kg
	Release	812 cm3	2.5 kg
Subtotal		376,262 cm3	875.7 kg
Hull		55,597 cm3	172 kg
Total		851 L	1047.7 kg

Submarine

Part	Subpart	Volume	Weight
Barometer		314 cm3	~50 g
Gyroscope		in computer	negligible
Accelerometer		in computer	negligible
Research equipment	Gas emission	1000 cm3	~2.5 g
	Camera x2 (each)	156.24 cm3	.1 kg
	Spectrometer	96 cm3	.1 kg
Power source (3000 W)	Shielding	586 cm3	9.09 kg
	Battery	1,900 cm3	1.8 kg
	Thermocouple	Thin films, negligible	50 g
Communications	Emitter	250 cm3	.2 kg
	Receiver
Method to sample water	Disk	1,000 cm3	3.1 kg
	Gears	1,000 cm3	1 kg
	Motor	4,500 cm3	4.5 kg
Lights		60,000 cm3	1 kg
Computer		1,000 cm3	3 kg
Propulsion	Shafts	760 cm3	2.36 kg
	Motor	4,000 cm3	3.5 kg
Subtotal		76,560 cm3	29.85 kg
Pressure-resistant hull		5 cm thick	173.1 kg
Propellers		25.6 cm3	79 g
Total		141 L	203.0 kg (202.7 for windows)

The calculated volume is the volume required if all components could be stacked without air in between them. Thus volume of the internal components has been increased by 10% to allow for more room of the internal components. That results in an internal volume of 85000 cm³, a sphere with a radius of 27.3 cm.

Digger parts list expanded on the back of an envelope

The calculated thermal power requirement for the digger was calculated to be around 1 MW, much more than the 10 kW for which the original heat source was designed. A 1 MW source of plutonium will have a mass of 1.79 metric tons, which also needs to be subcritcally encapsulated in lead. Furthermore, the increase in heat source volume will mean that the digger will have to dig a wider tunnel and will thus increase the power requirement. Needless to say that this will not be a viable option for the mission. To solve this problem, NASA is designing power systems for space missions that are able to provide this 1 MW (and more) for extended periods of time.[65] The selection of these systems, however, is considered outside the scope of this project. This is in part due to the complexity and in part due to the fact that these systems are still being optimised. The paper mentioned above discussed reactors that produce up to 15 MW of power with a mass of 2 metric tons. Scaling this down most likely won’t go linearly, but it will be assumed that the power scales with the square of the mass. Thus decreasing the power by a factor of 15 will reduce the mass to about .5 tons. Assuming that the reactor has half the density of plutonium, the reactor will have a volume of 60.6 L.

The digger will fix itself inside the ice using spikes that are pushed outward from within the digger. These spikes are pushed out by a system that is similar to a windable car jack. This setup will require a length of MMC equal to 1.5 times the circumference of the digger, with a diameter of 5 cm, which results in 7.5 L of MMC, or 22.95 kg. It will make the digger 5 cm longer.

The computer in the digger can be much smaller than in the submarine as it needs much less autonomy.

The cable is with the digger to avoid having to drag all that length of cable all the way through the ice, and to make sure that it’s possible to unroll the cable. Since the actual required thickness is almost impossible to test at this point, a round copper wire with a diameter of 1 mm shall be assumed. This will have a volume of 8.63 L. It will also require some room to unwind, so this will be increased to 10 L.

The balloons on the sides of the submarine can best be filled when the digger has breached the crust. This way the frontal area of the digger can be reduced, decreasing the power cost. However, this will require a filling system in the digger, making it longer. This will require a tank for the pentane; a cylinder with a radius of 30 cm and a volume of 105.3 L, so a length of 37.2 cm. Giving this tank a hull of 1 cm thick MMC, yields a total outer volume for the tank of 118 L, and a mass of 40.4 kg. Furthermore it will require a piston, say, a 2 cm thick disk and a 5 cm diameter driveshaft (6.5 L and 20.3 kg of MMC). Lastly it will require a strong motor capable of filling the balloons against the 10 MPa of pressure at the crust-sea border. This force can probably be achieved using gears, but the exact motor to use for this is difficult to determine. Hence a much larger motor than used for the inside of the submarine will be assumed. Tripling the volume and mass of the stronger motor yields: 12 L and 10.5 kg. Summing all this up yields 136.5 L and a mass of 147.1 kg.

The release system will mostly rely on a few hinges and some extra support to connect the upper and lower half of the digger. Probably 2 support beams as long as the diameter of the submarine and 4 cm in diameter will suffice. This is 812 cm³, 2.5 kg.

The hull will be made 1 cm thick with the MMC aforementioned many times: 6061 Al/SiC fibre UD. It will be a cylinder with a radius of 35 cm, capped off by a hemisphere with the same radius. The length of the cylinder can be determined by equating the volume of such a cylinder with length x to the internal volume of the digger (without the submarine, as it cannot be puzzled in with the rest of the equipment. This will result in a length increase of 65 cm), which results in x=37.8 cm. This 37.8 cm is the length of the cylinder if all components could be liquefied and poured into the submarine. This, however, is obviously not the case. Therefore the 3 components giving the largest contribution to the mass will be considered, and it will be assumed that the other components can be placed around those. The power source is needed most in front of the digger, where the ice should melt. Thus, it will be placed in the hemisphere in front and a little further down the shaft of the digger if needed. The hemisphere has a volume of 89.8 L, which is more than the power source volume. Thus the power source can be placed in the front of the digger, even with some room to spare.

The submarine requires 65 cm to be placed in the digger. The pentane tank requires 39.2 cm for the tank itself. Furthermore, the driveshaft requires the same length + some to spare, leading to a total of 90 cm. Lastly, there is the thickness required for the fixation spikes, 5 cm. Summing all this, gives a cylinder length of 160 cm.

The volume of the digger hull will then be: 4/3 ((35+1)3-353)+*160*((35+1)2-352)+*1*((35+1)2)=55.6L resulting in 172 kg of MMC. The external volume of the digger then becomes 851 L.

Submarine

Shape

In baseline 2 primary shapes would be possible for the submarine: A cylinder (or pill) and a sphere. The cylinder has the significant advantage that it has room for much more volume behind a much smaller surface area. For instance, a pill shape twice as long as it is wide has 150% more volume than a sphere with the same frontal area (π a^2 where a is the radius of the sphere). However, a pill shape is much more prone to lobar buckling than a sphere [66], which means that even though in theory a pill shape could be more streamlined than a sphere with the same hull thickness, a pill shape will either require internal structures to support it, or a thicker hull. Both will result in a larger total required volume (and thus more frontal area) and more mass (and thus more required propulsion), ultimately nullifying the added benefit of the pill shape.

Windows

Figure 9: schematic representation of the diamond windows embedded in the hull. Lengths are in mm.

The submarine needs windows for the cameras to be able to see through. The problem is that very few transparent materials have a yield strength over 300 MPa. Polycarbonate, for instance (which is infamous for being indestructible; it is also used for bulletproof glass) has a yield strength less than 100 MPa. However, as these are small windows rather than a full sphere, a diamond lens might actually be possible and affordable. For instance, a 40 mm diameter window, 13 mm thick, would be roughly 441 carat. One such windows is shown schematically in figure 9. Preferably the submarine has 2 of these windows, sharpened to be super smooth. An added benefit is the fact that diamond has a very high refractive index, which may make it possible to use the window as a fish-eye lens to allow greater view of the surroundings with the same number of cameras. 2 windows made of cut diamonds (which can be around 10 times more expensive than uncut diamonds) can turn out to be about 2.9 million USD.

These windows save some space and cost some other space at the same time. For instance, to avoid a structural weakness, the hull besides the window will be strengthened with extra material. This will be assumed to have the shape of a cone with a cylinder taken out of it. The cone has a base radius three times that of the window, and a height of 37.5 mm (This way the extra material reaches the thickness of the hull at the cutoff cylinder). The resulting volume is 104.7 mL. The gain is a cylinder 25 mm tall with a diameter equal to the lens diameter: 31.4 mL. The difference between these two is the net volume and mass loss: 73.3 mL, 227 grams of MMC.

Mass, pressure shielding and flotation

The part concerns itself with enveloping a given volume and mass of internal components in sufficient shielding material.

The total mass of the internal components is 77.2 kg. The total volume of the internal components then stops at 97 L. In a spherical configuration this results in an inner hull radius of 28.5 cm. To build a 15 cm steel hull (density 7840 kg/m^3) around that, requires 1942 kg of steel.

Since the maximum capacity of the Falcon Heavy rocket is 3.5 metric tonnes, and this 2 ton ball still needs to be encapsulated in a torpedo, held up by a landing base, this is too heavy. Thus lighter and stronger materials are taken into account, to reduce both the volume and the mass required.

Diamond could in theory be used, an added benefit is that it is easy for the cameras to see through. Diamond has a compressive strength of roughly 60 GPa. This is a factor of 10.4^2 more than steel. Taking this 10.4 as our thickness ratio, results in a diamond layer thickness of 1.436 cm. This would have a weight of only 54 kg. This is equivalent to 270,000 carat. At an uncut diamond price of $375 per carat, this results in a hull cost of about 100,000,000 USD. Furthermore, since diamond is very difficult to weld together, this would require a spherical diamond of the required volume; which then needs to be hollowed out. The costs of finding and cutting such a diamond are probably well beyond anything anyone would ever be willing to pay, if it is possible at all.

The solution is probably to use composite materials, specifically a Metal Matrix Composite: 6061 Al/SiC fibre UD. At a compressive strength of 3000 MPa (Nearly 5.5 times that of high-strength steel), much less material is required; a factor 3 decrease in the thickness of the material with respect to steel is assumed. Furthermore, it has a density of 2700 kg/m^3, a 66% decrease from steel. A 5 cm layer around a 28.5 cm sphere has a volume of .0605 m^3, which with a density of 2700 kg/m^3 will have a mass of 163.4 kg. The total density of the submarine is then 240.6/.1575=1528 kg/m^3

The formula to carry this mass with a solid buoy is m=^(m0-V0w) / _(w/-1). Where m and are the mass and density of the buoy respectively. The reason to use a solid is that solids can be fixed to the side of the submarine more easily. Liquid buoys require canvas to hold them in place, whereas solids simply require cables or glue.

Looking at the list of densities of various solids on engineering toolbox[67], to get an idea of densities of several solids, it is found that the main solids with densities lower than 1 kg/m³ can be subdivided into 2 categories: solids with air in them - like wools, snow or foams - and wood. The problem with the aerated solids is that they will either collapse under the pressure, or soak themselves with water. Both scenarios will cause the air to escape from them, leaving a much denser material behind.

This leaves the woods. Wood does also soak in water, but can be coated more easily. The lightest wood mentioned is balsa wood, at a density of 130 kg/m^3. Filling this in in the formula above gives 12.4 kg of balsa wood, which translates to a sphere with a diameter of 56.7 cm, or a cylinder with diameter and height 50.0 cm. However, the problem is that balsa wood already has trouble with pressures between 10 and 20 MPa (according to the following source[68]), well below the required 300 MPa.

Woods in general tend not to work all too well, as outlined by Bucur et al.[69] The problem is that although wood as a whole is rather strong, the cellular structure is not resistant to too high pressures, as the long cylindrical shells are at risk of lobar buckling. After extended periods of time under high pressures, this will compromise the structural integrity, causing the wood to fail entirely. Furthermore, the US forest service showed that very few woods (used commercially in the united states) have a compressive strength higher than 100 MPa, and the ones that do (Kaneelhart and Macawood) have a density of 94% that of water.[70] That density would require 1302 kg of wood. Furthermore, this compressive strength only works along the grain. Perpendicular to the grain they too would already fail just below the Europan surface.

Instead, one can also use a watertight balloon filled with a light liquid for flotation.[71] One can for instance use pentane, which has a density of 626 kg/m^3. This will require 139 kg of pentane; i.e. a sphere with a radius of 37.6 cm. If one is attached to the top and one is attached to the bottom of the submarine (to remove the orientations up and down from the submarine), each could have a radius of 29.8 cm, smaller than the submarine.

Then there are 2 more options for automatic flotation: A ‘steel balloon’ (not necessarily made out of steel), or a larger submarine. The benefit of increasing the submarine size, is that the hull has the most efficient distribution of volume; one sphere has less area per unit volume than 2 spheres. That means that less material will be required to make the hull. However, two spheres can be aligned inside the digger, to allow for a slimmer, longer digger, which would require less power to dig through the crust and less material to build. If increasing the submarine size, the radius of the vessel that will result in an average density equal to water must be determined.

The density of the vessel will be: ρ=(m0+4/3 π*3000 {(R+.05)³-R³})/(4/3 π R³), using the MMC mentioned above. R is the outer radius of the hull. Then ρ=1000 kg/m³ is set, solve for R and find: R=50.1 cm. The mass of the hull will then be 428.4 kg. In practice, it should be taken into account that a larger hull radius will require a thicker (and thus heavier) hull as well, which should thus be larger and therefore thicker, etc. For an MMC balloon, the above formula transforms into =(m0+4/3 *3000 (R3-(R-.05)3)/(V0+4/3 R3) V0 is equal to the .1575 cubic metres of the submarine. Solving for R gives: R=43.9 cm. The mass of the balloon is 405.5 kg.

In the first case, the total mass will be about 505 kg. The second case will yield a 645 kg balloon. This shows that a larger submarine will have a significant mass advantage over a ballooned submarine. Using the idealised case of diamond would yield a 34.9 kg balloon with a radius of 30.4 cm (costing 65 million USD) giving a total of 275 kg. A full diamond hull allowing flotation would give the total a mass of 265 kg, but the hull would cost over 300 million USD.

In conclusion, if the submarine should be made to float, this cannot be done with a solid buoy, as virtually all materials light enough are too weak or porous. The two hull solutions will both result in immense masses, unless a material like diamond could be used.

The best solution is thus to use 2 balloons filled with pentane. They can be attached either to the top and bottom or the sides of the submarine. This will ensure that it is balanced in its buoyancy, so that it can still turn in every direction, and keep it afloat with a reasonable mass.

Engines

Figure 10: The buoyant force on the submarine as function of depth for different masses of pentane balloons. The lowest curve is for 20 kg of pentane.

The 4 engines should be able to provide sufficient lift at all times to keep the submarine afloat. The specific volume of pentane has been determined based on data collected by Paul Carle [72]. Based on the data the specific volume as function of pressure was determined to be V/V0=exp(3.92*10^-5p) where p is the pressure in MPa and V0 is the volume at p=0. With the current (unadjusted) volume for the submarine and the chosen balloon type, the total force (gravitational + buoyant) on the submarine as function of height can be calculated for various balloon masses. Figure 10 shows the total force as function of depth (left is the seafloor) for balloon masses of 20, 40,... through 120 kg, with 20 kg being the lowest curve.

Now, the choice for the balloon mass can be made in one of two ways: One may consider the total energy required to get to the seafloor and choose a mass that minimizes that. For instance, of the 6 chosen masses this total energy will be lowest for 80 kg. However, as the graph shows, the 80 kg balloon will result in a net buoyant force on the seafloor, which is where the submarine will stay for the longest time, which means that the submarine will have to supply power continuously for the largest part of the mission. Alternatively one may choose a balloon mass which ensures that the buoyant force on the submarine is zero on the seafloor. This mass would for these parameters be 65 kg. An additional advantage is that at this balloon mass the submarine sinks until it reaches the seafloor. The result is that to get down to the seafloor, no power has to be used. During the journey down power is only required to stop for pictures or measurements or to move laterally. This latter choice seems most convenient, in which case the maximum net force to be overcome is -40 N. To allow upward sailing as well, the minimum total thrust the submarine should be able to provide should be well above that; about 400 N.

Blanke et al. [73] made an analytical model that determines the produced thrust and required torque for rotors. From this model, the rotor dimensions and the motor power will be determined. The model states that the thrust delivered by a single propeller is equal to T=d⁴*a0*n²-d³*a1*n*u and the torque required to drive it Q=d⁵*b0*n²-d⁴*b1*n*u With T the thrust in N, Q the required torque in Nm, ρ the density of the medium, d the diameter of the propeller, n the angular frequency of the propeller in rad/s and u the water velocity in m/s. a0, a1, b0 and b1 are dimensionless coefficients. a1 and b1 have been determined to be smaller than 0, meaning that as the submarine picks up speed, the thrust will go up. (So does the required torque, but because the thrust increases we can reduce the propeller speed at higher velocities to reduce the torque a little again.) Thus, we will do the following calculations from standstill (u=0). Furthermore, ρ=1000, because at that point the propellers will have to give the most thrust since the buoyant force is lowest. With 4 propellers, the required thrust per propeller is 100 N. We can fill this in in the equation for T to find the propeller diameter that - given a certain propeller velocity - will result in a thrust of 100 N. The solution is: d=(10/n)^0.5*(ρ a0)^1/4=0.688311*n^-0.5 The reason to do it this way is because the velocity is usually a constraint of the motor, whereas the propeller size can be increased almost indefinitely. This expression can then be inserted into the equation for Q, to find Q as function of n or d: Q=10^5/2*ρ^-1/4*b0*a0^-5/4*n^-1/2=10.1551*n^-0.5=100 b0/a0*d=14.7537 d With these equations it is possible to uniquely determine for a maximum torque constraint what propeller velocity and diameter are required, or for a maximum velocity constraint what minimum torque is required. As an example, the following electromotor will be used: [74] with a maximum torque of 2 Nm and maximum rotational speed of 2730 rpm (286 rad/s). Hitting the maximum torque of 2 Nm will require 13.6 cm propeller blades spinning at 25.782 rad/s (9% of the maximum speed). Hitting the other end of the spectrum, 286 rad/s, will require .6 Nm of torque on 4.07 cm blades (30% of the maximum torque). Using gears, it is possible to drive all 4 propellers with a single motor. However, at the high-velocity limit, the total torque required to drive all 4 propellers is 0.6*4=2.4 Nm, which the motor can’t supply. The other end, however, can be achieved. If the propellers spin at 25.78 rad/s, each propeller requires 2 Nm to spin. However, if the transmission to the propellers is 5:1, the motor needs to supply only 0.4 Nm of torque per propeller. It has to spin 5 times as fast, but that will be about 128.9 rad/s, well below the constraining 286 rad/s.

This particular motor requires 180 W of power to run. In terms of mass and volume, this setup requires 4 gears and driveshafts, one worm drive and 4 propellers with 6.8 cm blades. All this can be made from the MMC as well, as it is relatively lightweight and very strong. 20 tooth steel gears are available at around 40 grams and 120 cm³, worm drives at similar sizes. The shafts should have diameters around 1 cm and lengths of R+d/2=50 cm, resulting in about 40 cm³. The propeller blades will be approximated with ellipses 2 mm thick, and half axes 3.4 and 1 cm. 12 of them gives a total volume of 25.6 cm^3. 120*5+40*4+25.6=785.6 cm³ of MMC, giving 2.44 kg. Then we add this to the volume and mass of the electromotor: 4 L and 3.5 kg. This means that for propulsion we require an additional 5.94 kg, and 4.79 L. Bear in mind that of this 4.79 L, 0.025 is outside the submarine.

Power requirement

The total power requirement is then determined by a strong computer with no screen, 2 cameras, 1 motor and some to spare for the water inlet. For the computer we will make an assumption based on the power requirement of the HP zbook computers used by students at the TU/e. The chargers of these computers require 150 W of power, but this power is used to power the screen and charge the battery as well. Since the on-board computer of the submarine won’t have any use for a screen and can be supplied with power continuously (thus it does not need extra power to charge the battery even when it is running), we will assume a power of 50 W for the computer. Camera chargers use powers in the order of 5 W [75]. The motor uses 180 W. The total is then 240 W. We will increase this by 25% to compensate for deviations and to charge the battery for the slot sampler. We will then require a total of 300 W. With an RTG efficiency of around 10%, that requires a 3000 Wth power source by the end of the mission. Accounting for the decay of plutonium, the initial power required would be 3300 Wth, which would weigh 5.89 kg (still below the critical limit) with a volume of .304 L. This is equivalent to a sphere with a radius of 4.17 cm. To shield this with the 1.02 cm to avoid damage to the on-board computer will result in a sphere with a radius of 5.19 cm and a volume of 586 cm³. The mass of the shield will be 3.20 kg, resulting in a combined mass of 9.09 kg. The size of the thermocouple has so far been neglected as thermocouples can be made in the form of thin films, 2 μm thick [76], which even for a heavy metal like plutonium would have a mass of 50 grams. The precise metals for the thermocouple are not known yet, but by selecting plutonium for both metals, it can be ensured that the mass will definitely not be underestimated.

Radiation resistance of experimental equipment

Cameras resistant to radioactive radiation are easily available, such as the one on the following site.[77] However these are a lot larger than for instance the gopros mentioned before. Wang et al.showed that regular HD cameras show some deterioration after exposure to radiation.[78] Camera sensitivity decreases up to 70% at dose rates of 1000 rad/h during 8 hours exposure. However, due to shielding it will be less than 1 mrad/h. That means that the total dose of 8000 rads will be reached after 8 million hours, i.e. 900 years. The camera will be exposed to less than 1/50th of that radiation over the course of the mission. Furthermore, the fact that the radiation intensity is much lower may ensure that the camera is not damaged at all. That means that in regards to the camera, the radiation will not be a problem. Spectrometric experiments usually require a correction[79], but this can be applied to the measurements after the fact and does not need any additional shielding. Integrated circuits do require shielding from radiation, as high-energy gamma photons can excite electrons and cause signal spikes, as explained by Yu et al.[80] For that, an additional shielding for the integrated circuits is proposed.

Submarine grease maintenance

Submarines have their grease applied quarterly when they are in port.[81] This grease is applied to several locations on the outside of the hull of the submarine, specifically locations where there are moving parts on the outside. Since no person will be present on Europa to apply and refresh the grease on the submarine, it is a necessity to ensure that the submarine is greased well enough to endure a very long time in a very cold saltwater environment.

Conclusion

Out of all the components of the design, the only aspect that might turn out to make the mission impossible is the transmission of acoustic signals through water. However, even for this there are caveats. For the rest, the submarine, the digger and surface lander can presumably be built with ‘simple’ components; either devices readily available on the internet (such as the motors), or materials composed of only one or 2 elements (such as copper or PuO₂) rather than convoluted compounds. Using these simple components, a design has been presented with which the mission can be brought to fruition within the range of the requirements and constraints. As a space agency like NASA presumably has access to more complicated components, they can probably fulfill the requirements of the mission with even less difficulty. So to conclude, the basis for a robot has been designed which can land on Europa, dig through the icy crust and send a submarine into the sub-surface ocean to search for life, signs of life or conditions that may support life in or on Europa. With this, the goal of this research has been completed.

Discussion

Hydrophobic coatings in viscous media

Something to take into consideration is the fact that at high pressures, the viscosity of water increases slightly. As the viscosity increases, shear stresses on external components of the submarine will increase as the submarine goes deeper down. This may decrease the time scale over which coatings on electrical equipment deteriorate and wear down. However, the magnitude of this effect can only be determined sufficiently with modelling software unavailable to us. Hence this will just be left as something to consider for others to take into account when continuing with the design.

Opening of the digger and releasing the submarine

To reduce the diameter of the digger (and thereby its power requirement) as much as possible, the submarine will sit in the digger with the propellers folded down. This of course requires the propellers to fold up upon releasing the submarine. The precise workings of this were not discussed in detail, but here a brief outline of the general idea to get the propellers in position will be proposed. The digger opens up like a flower, in 4 sections of hull which fall outward. The submarine is then released before the balloons have been inflated (so that the submarine will sink). This way, the propellers will land on the hull sections, so that they will be pushed up and lock in place by the sheer force of gravity on the submarine. After that, the submarine will be able to sail away.

Absorption of sound waves by salt water

Multiple papers have researched the absorption of sound waves by salt water [82][83][84][85]. The cited papers correlate the absorption of sound waves by sea water to sound frequency, water temperature, depth, and other factors. However, these papers state that their calculations are only accurate for water that has a temperature higher than -2, 0, or -6 degrees Celsius. Since the water on Europa is most likely colder than -6 degrees, using the models described in these papers would yield significantly inaccurate results. The composition of the ocean of Europa is furthermore unknown, and the salinity of water is another factor that sound absorption is correlated to. This is simply a problem that requires more research.

The effect of temperature and pressure on the functioning of the surface lander

Many STP liquids are solid at Europan temperatures but will due to the low pressure sublimate immediately. Fluids can most likely only be kept in pressurised containers. As the surface lander was not the main focus of this research, here only a few considerations for future research will be mentioned. The low pressure can pose a significant problem concerning the cooling of the surface base, as there will be little air to lose heat to. The air will be super cold to create a significant temperature differential, but super thin as well which reduces conductive cooling. Furthermore, the low air temperature reduces convective cooling. If gains and losses are properly balanced, it might actually be possible to keep the body of the vehicle at a significant temperature with the thermonuclear battery heating it up.

Appendix

Planning

Week 1:

• General research: we explore the topic that we chose and come up with inspiration for a research objective. (Kasper, Marco, Wouter)
  • Deliverables: potential research objectives
• Define users: according to the topic we have chosen, define the users that play a role in achieving this objective. (Kasper, Marco, Wouter)
  • Deliverables: a list of users and their goals with regards to this research

Week 2:

• Determine objective: from the research in week 1, determine a research objective that will be the center of your research. (Kasper, Marco, Wouter)
  • Deliverables: definitive research objective
• Determine requirements for the research objective and its users: in order to achieve an answer to the research objective, what requirements should there be, from the standpoint of the users (Kasper, Marco, Wouter)
  • Deliverables: a list of users and the requirements that they have for this research objective
• Planning: make a planning for the remainder of the course (Kasper)
  • Deliverables: a planning of things that are still to be done for this research
• Consult NASA/ESA: contact NASA and the ESA for their take on how to go about designing a research mission (Marco)
  • Deliverables: advice from NASA/ESA to improve our research

Week 3:

• More extensive user descriptions: further work out what users would be involved with our research objective (Wouter)
  • Deliverables: a more elaborate description of the users and their requirements with regard to the research objective
• More extensive planning: work out the planning of the project in more detail. What should be done when and by who, with which deliverables (Marco)
  • Deliverables: a more detailed planning for the remainder of the course
• Examine possible experiments on Europa: research for different experiments that can be done on Europe to examine our objective. (Marco)
  • Deliverables: Some experiments that can possibly be executed
• Work out section 3.2: research whether it is feasible and worthwhile to perform research below the surface of Europa (Kasper)
  • Deliverables: a section on whether it is feasible to go below the icy surface of Europa, and, if so, what kind of research to perform there and how to do it.

Week 4:

• Investigate the communication line: How to establish a stable communication between Earth, the lander on Europan surface and the submarine in the ocean (Marco + Kasper)
  • Deliverables: a description of the possible technologies for this communication line
• Update the user descriptions: Elaborate the requirements, preferences, constraints and measurability of the requirements and constraints (Wouter)
  • Deliverables: A clear list of the user requirements, preferences, constraints and measurability of the requirements and constraints
• Update the experiments: Finalize the experiments that we want to execute on Europa. (Marco)
  • Deliverables: A clear list of experiments that needs to be executed during the mission
• Work out the rest of chapter 2: describe the possible problems that Europan physics pose to the mission, along with possible solutions or workarounds (Wouter)
  • Deliverables: a list of potential Europan physics challenges, along with solutions/alternatives for them
• Presence of a sub-surface ocean: search for the indications/proof of a sub-surface ocean
  • Deliverables: A clear section about the indications/proof of a sub-surface ocean
• What to do with extraterrestrial life: Elaborate the way we should adapt the mission the the possible extraterrestrial life on Europa (Kasper)
  • Deliverables: A description of the dangers of the mission and possible solutions/adaptations that has to be made

Week 5

• As week 4: continue to work out the chapters that everyone was assigned to (Kasper, Marco, Wouter)
  • Deliverables: finish the work everybody started with
• Duration of the mission: Examine how long the mission needs to last (Wouter)
  • Deliverables: An estimation of the duration of the mission
• Parts list: Make a parts list of the submarine, digger and lander (Wouter)
  • Deliverables: A clear and complete parts list for every part of the robot
• Model for digging through ice: Make a model for digging through ice, how long this will take and how much power is required.” (Wouter)
  • Deliverables: How long is the digging going to last with how much power
• Which power source: Investigate which power source is most suitable for this mission (Wouter)
  • Deliverables: A suitable power source for this mission
• Where will the experiments be performed: Elaborate where all the experiments will be performed (Marco)
  • Deliverables: A clear description of all the experiments and where they will be performed
• Structure: Make a clear structure for the report and see what is missing (Kasper)
  • Deliverables: A clear structure which we can hold on to during the remaining time of the project

Week 6

• As week 5: continue to work out the chapters that everyone was assigned to (Kasper, Marco, Wouter)
  • Deliverables: finish the work everybody started with
• Solve water problems: Investigate what possible solutions are for some problems in the high-pressured water, like navigation, floating and taking water samples (Wouter + Marco)
  • Deliverables: Proposals for a way to navigate, float and taking water samples in the Europan water
• ”Determine the autonomy:” State what level of autonomy the robot must have (Kasper)
  • Deliverables: A clear description of the robots autonomy
• Structerise introduction and communication: Combine all different parts that are written and complement these parts into a complete section (Kasper)
  • Deliverables: A finished introduction and communication section

Week 7

• As week 6: continue to work out the chapters that everyone was assigned to (Kasper, Marco, Wouter)
  • Deliverables: finish the work everybody started with
• The final robot: Constitute the submarine, the digger and the lander based on the parts list (Marco en Wouter)
  • Deliverables: A clear description of the submarine, the digger and the lander
• Technical sketch of the lander: make a technical sketch of the lander that showcases in detail what it is expected to look like (Marco)
  • Deliverables: technical sketch
• Create presentation: create a presentation, to be given in week 8 (Kasper, Marco, Wouter)
  • Deliverables: presentation

Week 8

• Write conclusion and discussion: write a conclusion that describes the findings of our research, and a discussion that describes what could have been done better/differently (Kasper, Marco, Wouter)
  • Deliverables: a conclusion and a discussion in the report
• Presentation: present our report to the tutors of the course (Kasper, Marco, Wouter)
  • Deliverables: a presentation for a fantastic grade
• Finalize wiki: make sure the wiki is up to date with all our findings and the report that we have written (Kasper, Marco, Wouter)
  • Deliverables: an up-to-date wiki

Calculations

As we appeared unable to use the wikimedia math markup, many of the calculations are very difficult to decypher in the text. For that, we will place here the download links for the wolfram mathematica files that contain all calculations. They have for the most part been annotated to be legible.

TU/e students and employees can download mathematica and the licenses here.