Viotar/ Ways To Exite The String: Difference between revisions
Jump to navigation
Jump to search
No edit summary |
No edit summary |
||
| (18 intermediate revisions by 3 users not shown) | |||
| Line 1: | Line 1: | ||
{|cellpadding="10" | {|cellpadding="10" | ||
|-valign="top" | |-valign="top" | ||
|width="100%" style="border:1px solid #fabd23; background-color:#FEF5DE;"| | |width="100%" style="border:1px solid #fabd23; background-color:#FEF5DE;text-align: justify;"| | ||
{| padding="0" cellspacing="0" style="margin-top:.2em; width:100%; background:none" | {| padding="0" cellspacing="0" style="margin-top:.2em; width:100%; background:none" | ||
| rowspan="32" width="5px" | | | rowspan="32" width="5px" | | ||
| Line 24: | Line 24: | ||
'''Subpages:''' | '''Subpages:''' | ||
</h1><br/> | </h1><br/> | ||
{{:Viotar_Menu}} | |||
|width="2%" style="background-color:#FEF5DE;"| | |width="2%" style="background-color:#FEF5DE;"| | ||
|width="49%" style="border:2px solid #fabd23;"| | |width="49%" style="border:2px solid #fabd23;text-align: justify;"| | ||
<h1 style="font-size:180%; border:none; margin:0; padding:0"> | <h1 style="font-size:180%; border:none; margin:0; padding:0"> | ||
'''Overview:''' | '''Overview:''' | ||
</h1><br/>There are several | </h1><br/>There are several possible ways to excite the string of the Viotar. The only two criteria are that a Helmholtz vibration is reached and that the Viotar remains playable as a normal guitar.<br/> | ||
Four possible ways to excite the strings have been chosen for further research. The main focus of this research will be on feasibility of the construction, on whether Helmholtz can be achieved and if it’s possible to meet the design requirements. | |||
The four possibilities that will be researched are: | |||
The four | |||
* Electro-magnet | * Electro-magnet | ||
* Piëzo-element | * Piëzo-element | ||
* Bowing wheel | * Bowing wheel | ||
* Bowing belt | * Bowing belt | ||
The findings of these researches will be listed below, from the four possible ways the most feasible will be chosen for further research.<br/> | |||
The findings of | |||
<br/> | |||
|}<br/> | |}<br/> | ||
{| cellpadding="10" | |||
==Exitation by Electromagnet== | |-valign="top" | ||
===Principle=== | | style="border:2px solid #00FF33; background-color:#CCFF99"| | ||
==Ways to exite the string== | |||
===Exitation by Electromagnet=== | |||
====Principle==== | |||
Another way to exite the string is by using an electromagnet force on the steel strings. This force is created by the flow of current through a coil wrapped around a magnetizable core, creating an electro magnet. The force can be controlled by changing the current that flows through the coil. Because current can be controlled by speeds in the order of several Gigahertz, the flow can be controlled very rapidly. | Another way to exite the string is by using an electromagnet force on the steel strings. This force is created by the flow of current through a coil wrapped around a magnetizable core, creating an electro magnet. The force can be controlled by changing the current that flows through the coil. Because current can be controlled by speeds in the order of several Gigahertz, the flow can be controlled very rapidly. | ||
| Line 73: | Line 56: | ||
The force in y-direction is calculated with: | The force in y-direction is calculated with: | ||
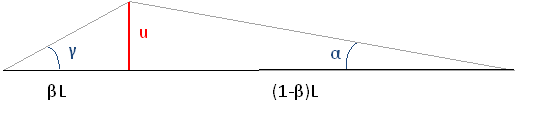
<math>F_y=sin(\gamma)A\sigma_{1}+sin(\alpha)A\sigma_{2}</math> | |||
<math>\sigma=E\varepsilon</math> | |||
<math>\varepsilon= {dL\over L_0 }</math> | |||
<math>F_y = AE \left (sin(\gamma){\sqrt{\beta^2 L^2+u^2}-\beta L \over \beta L} + sin(\alpha){\sqrt{(1-\beta)^2 L^2+u^2}-(1-\beta) L \over (1-\beta) L} \right)</math> | |||
The biggest static force acting on a string is the force when a short, thick string is exited. It is assumed that the length of this string is 20 cm and the diameter is 0.25mm. | The biggest static force acting on a string is the force when a short, thick string is exited. It is assumed that the length of this string is 20 cm and the diameter is 0.25mm. | ||
| Line 83: | Line 73: | ||
This gives the following for the part βL: | This gives the following for the part βL: | ||
[ | <math>F = {ma}</math> | ||
<math>F = \sum_{i=1}^{n}{m_ia_i}</math> | |||
<math>F_1 = \sum_{i=1}^{n}{m_i\left( {da \over dx} x_i \right)} = \sum_{i=1}^{n}{m_i\left( {a \over \beta L} x_i \right)}</math> | |||
<math>m_i = {\rho {\beta L \over n} A_{cross.sect.}}</math> | |||
<math>n = {x \over dx} = {\beta L \over dx}</math> | |||
<math>m_i = \rho A_{cross.sect.} dx</math> | |||
<math>F_1 = \int_0^{\beta L} {{a A_{cross.sect.} \rho} \over {\beta L}} x \;dx</math> | |||
<math>F_1 = {{a A_{cross.sect.} \rho} \over {\beta L}} \int_0^{\beta L} x \;dx</math> | |||
<math>F_1 = {{a A_{cross.sect.} \rho} \over {\beta L}} [ \frac{1}{2} x^2 ]_0^{\beta L} </math> | |||
And for the part (1-β)L:<br/><br/> | And for the part (1-β)L:<br/><br/> | ||
[ | |||
<math>F_2 = {{a A_{cross.sect.} \rho} \over {(1-\beta) L}} [ \frac{1}{2} x^2 ]_0^{(1-\beta) L} </math> | |||
Where: | Where: | ||
a = The acceleration of the string on position βL<br/> | a = The acceleration of the string on position βL<br/> | ||
A<sub>cross.sect.</sub> = The cross section area of the string <br/> | |||
ρ = The density of the string <br/> | ρ = The density of the string <br/> | ||
The total force needed to accelerate the string is given by:<br/> | The total force needed to accelerate the string is given by:<br/> | ||
[[ | |||
<math>F_{tot} = F_1 + F_2 </math> | |||
<math>F_{tot} = {{a A_{cross.sect.} \rho} \over {\beta L}} [ \frac{1}{2} x^2 ]_0^{\beta L} + {{a A_{cross.sect.} \rho} \over {(1-\beta) L}} [ \frac{1}{2} x^2 ]_0^{(1-\beta) L} </math> | |||
This gives a total acceleration force of 6,25N when the following parameters are used: | This gives a total acceleration force of 6,25N when the following parameters are used: | ||
a = 500 m/s2<br/> | a = 500 m/s2<br/> | ||
A<sub>cross.sect.</sub> = 5 ∙ 10-6 m2<br/> | |||
ρ = 8 ∙ 103 kg/m3<br/> | ρ = 8 ∙ 103 kg/m3<br/> | ||
| Line 106: | Line 122: | ||
This means that the maximum magnetic force that is needed is about 50 N. | This means that the maximum magnetic force that is needed is about 50 N. | ||
===Feasibility=== | ====Feasibility==== | ||
The calculation shows that a magnetic force of 50 N should be enough to exite the string in the desired pattern. Finding an electromagnet that generates this magnetic force is not a problem. However, the width of these electromagnets could be a problem, since there might not be enough space to place an electromagnet for each string separately. This problem could be solved by making the electromagnets on our own. The force that is generated in an electromagnet is only depending of the number of windings and the current through the coil. Since there is enough space underneath the strings, a long and tight electromagnet would work. | The calculation shows that a magnetic force of 50 N should be enough to exite the string in the desired pattern. Finding an electromagnet that generates this magnetic force is not a problem. However, the width of these electromagnets could be a problem, since there might not be enough space to place an electromagnet for each string separately. This problem could be solved by making the electromagnets on our own. The force that is generated in an electromagnet is only depending of the number of windings and the current through the coil. Since there is enough space underneath the strings, a long and tight electromagnet would work. | ||
Another point is that the magnet of one string might interfere with another and disturb it’s magnetic field. However, this problem would be quite easy to deal with as will since a screen could be placed in between the strings or the control device a magnet could compensate for disturbances from the others. | Another point is that the magnet of one string might interfere with another and disturb it’s magnetic field. However, this problem would be quite easy to deal with as will since a screen could be placed in between the strings or the control device a magnet could compensate for disturbances from the others. | ||
===Drawbacks=== | ====Drawbacks==== | ||
Despite the theoretical possibility to use electromagnets for actuation, there are two very important drawbacks in this concept. Firstly, the instrument will no longer be bowed although it sounds as such. In fact the instrument then will no longer be a bowing instrument, which was one of the main requirements. But probably the biggest disadvantage of this concept, is the quite illogical way of synthesizing the sound. To get the string in the desired vibration, this vibration should be known in advance. Getting this vibration would be no problem, since we can get it out of the model or simply record it from a real bowing instrument. But once the vibration is known, it has to be exited on the string using electromagnets, recorded using a sensor and then amplified before it is played. This is very strange since we already had the desired vibration when we started, and thus were already able to play it. | Despite the theoretical possibility to use electromagnets for actuation, there are two very important drawbacks in this concept. Firstly, the instrument will no longer be bowed although it sounds as such. In fact the instrument then will no longer be a bowing instrument, which was one of the main requirements. But probably the biggest disadvantage of this concept, is the quite illogical way of synthesizing the sound. To get the string in the desired vibration, this vibration should be known in advance. Getting this vibration would be no problem, since we can get it out of the model or simply record it from a real bowing instrument. But once the vibration is known, it has to be exited on the string using electromagnets, recorded using a sensor and then amplified before it is played. This is very strange since we already had the desired vibration when we started, and thus were already able to play it. | ||
===Conclusion=== | ====Conclusion==== | ||
The conclusion about this concept is that it would work most likely and that every vibration pattern could be chosen. However, the big drawbacks that it is no longer a bowing instrument and that the synthesizing routine is very illogical, make the concept fail. | The conclusion about this concept is that it would work most likely and that every vibration pattern could be chosen. However, the big drawbacks that it is no longer a bowing instrument and that the synthesizing routine is very illogical, make the concept fail. | ||
==Actuation by piëzo crystals== | ===Actuation by piëzo crystals=== | ||
===Principle=== | ====Principle==== | ||
A piezo actuator utilizes the phenomenon that crystals of certain substances expand when a voltage is applied on it or conversely, they give a voltage when pressure is exerted on the crystal. The name piezo is derived from the Greek word piezein, which means pressing. The concept for actuation by the piezo, is that the string is attached to a piezo element, which expands when a voltage is applied. The idea is that by varying the voltage, the movement of the string can be controlled, giving the desired vibration in the string | A piezo actuator utilizes the phenomenon that crystals of certain substances expand when a voltage is applied on it or conversely, they give a voltage when pressure is exerted on the crystal. The name piezo is derived from the Greek word piezein, which means pressing. The concept for actuation by the piezo, is that the string is attached to a piezo element, which expands when a voltage is applied. The idea is that by varying the voltage, the movement of the string can be controlled, giving the desired vibration in the string | ||
===Feasibility=== | ====Feasibility==== | ||
If the feasibility of this concept is considered, the combination of required force and deflection seems to be a problem. The reason is that piezo elements only enlarge a little, varying from a few micrometers to some tens of micrometers. There are piezo elements on the market that serve as a linear motor and have a maximum amplitude up to 100 mm, but again does not provide the required force. Another possibility is to use so-called piezo stacks. This a series of piezos allowing the maximum deflection to increase. However, this excursion is also just a little more than 1 mm for the required force, which is not enough. | If the feasibility of this concept is considered, the combination of required force and deflection seems to be a problem. The reason is that piezo elements only enlarge a little, varying from a few micrometers to some tens of micrometers. There are piezo elements on the market that serve as a linear motor and have a maximum amplitude up to 100 mm, but again does not provide the required force. Another possibility is to use so-called piezo stacks. This a series of piezos allowing the maximum deflection to increase. However, this excursion is also just a little more than 1 mm for the required force, which is not enough. | ||
===Drawbacks=== | ====Drawbacks==== | ||
The main disadvantage of piezo actuation is that the deflection they give is not large enough. This problem is partly dealt with using a stack, but even then the deflection is not large enough to make the string vibrate in the desired shape. Furthermore, in this design the instrument will no longer be a bowing instrument | The main disadvantage of piezo actuation is that the deflection they give is not large enough. This problem is partly dealt with using a stack, but even then the deflection is not large enough to make the string vibrate in the desired shape. Furthermore, in this design the instrument will no longer be a bowing instrument | ||
===Conclusion=== | ====Conclusion==== | ||
From the foregoing it can be concluded that it is not possible to get the string in the desired vibration using piezoelectric actuators. Also the instrument would not be a bowing instrument anymore, which is an important factor in the design. | From the foregoing it can be concluded that it is not possible to get the string in the desired vibration using piezoelectric actuators. Also the instrument would not be a bowing instrument anymore, which is an important factor in the design. | ||
==The bowing wheel== | ===The bowing wheel=== | ||
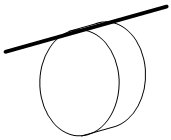
[[File:boww.PNG|frame|Border| Figure 1.1: Simple drawing of the bowing wheel with the string.]] | [[File:boww.PNG|frame|Border| Figure 1.1: Simple drawing of the bowing wheel with the string.]] | ||
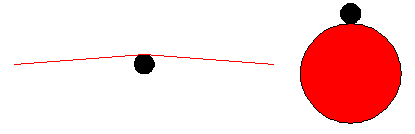
[[File:boww1.PNG|frame|Border| Figure 1.2: In both drawings the black dot is the cross section of the string and the bowing medium is red. Left: with a bow the bow hairs tilt slightly towards the string around the bowing point. Right: the surface of the bowing wheel tilt away from the string around the bowing point.]] | [[File:boww1.PNG|frame|Border| Figure 1.2: In both drawings the black dot is the cross section of the string and the bowing medium is red. Left: with a bow the bow hairs tilt slightly towards the string around the bowing point. Right: the surface of the bowing wheel tilt away from the string around the bowing point.]] | ||
| Line 161: | Line 177: | ||
This effect could hypothetically be prevented by the controller for the z-translation of the wheel. If the current friction force and normal force between the wheel and the string could be determined at every moment, the controller could “make sure” that these two stay at “natural” values (i.e. the same as when using a bow). This requires a constant reference for these both forces over the course of one vibration period, and a construction together with an actuator capable of following this reference with a total delay time including the sampling of the force measurements t_d that is much smaller than one vibration period, t_d≪T. This in addition with the sensors that are needed to measure the forces, makes bowing wheel a very unrealistic concept. | This effect could hypothetically be prevented by the controller for the z-translation of the wheel. If the current friction force and normal force between the wheel and the string could be determined at every moment, the controller could “make sure” that these two stay at “natural” values (i.e. the same as when using a bow). This requires a constant reference for these both forces over the course of one vibration period, and a construction together with an actuator capable of following this reference with a total delay time including the sampling of the force measurements t_d that is much smaller than one vibration period, t_d≪T. This in addition with the sensors that are needed to measure the forces, makes bowing wheel a very unrealistic concept. | ||
Latest revision as of 14:12, 5 April 2011
|