Mobile Robot Control 2020 Group 2

Group members

Marzhan Baubekova - 1426311

Spyros Chatzizacharias - 1467751

Arjun Menaria - 1419684

Joey Verdonschot - 0893516

Bjorn Walk - 0964797

Bart Wingelaar - 0948655

Design Document

Design document for the escape room challenge can be found here:

File:Mobile Robot Control Design Document Group2.pdf

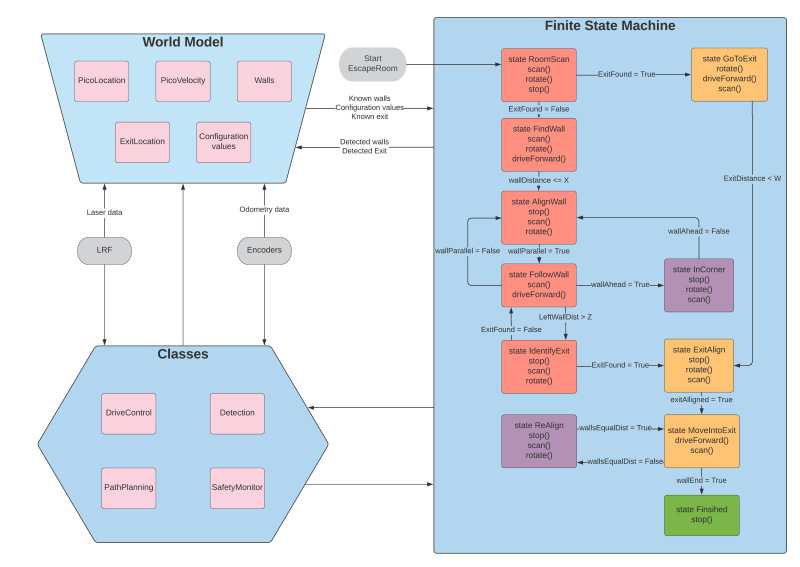
Escape Room Challenge

Path Planning

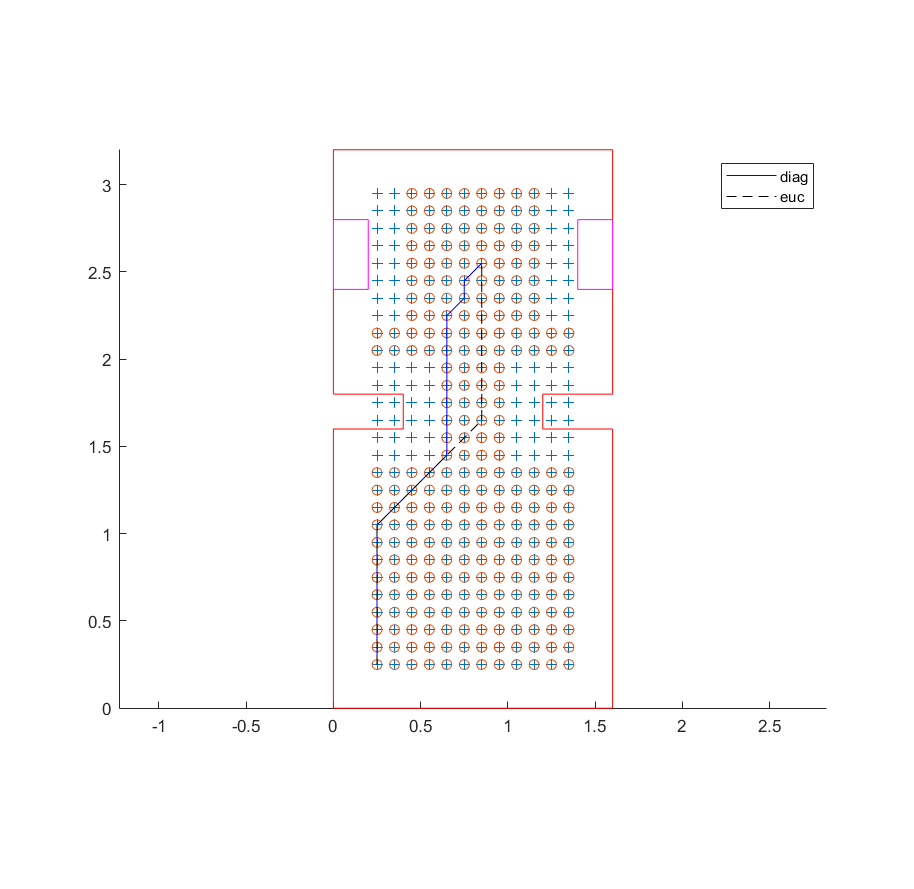
The algorithm that is chosen for the path planning is an A* algorithm. Before proceeding to algorithm, it is essential to transform map to a binary grid map. This step is done in Matlab and later translated to C++. There are two heuristic functions which are tested, namely diagonal and euclidian. Pathplanning with 2 heuristics.

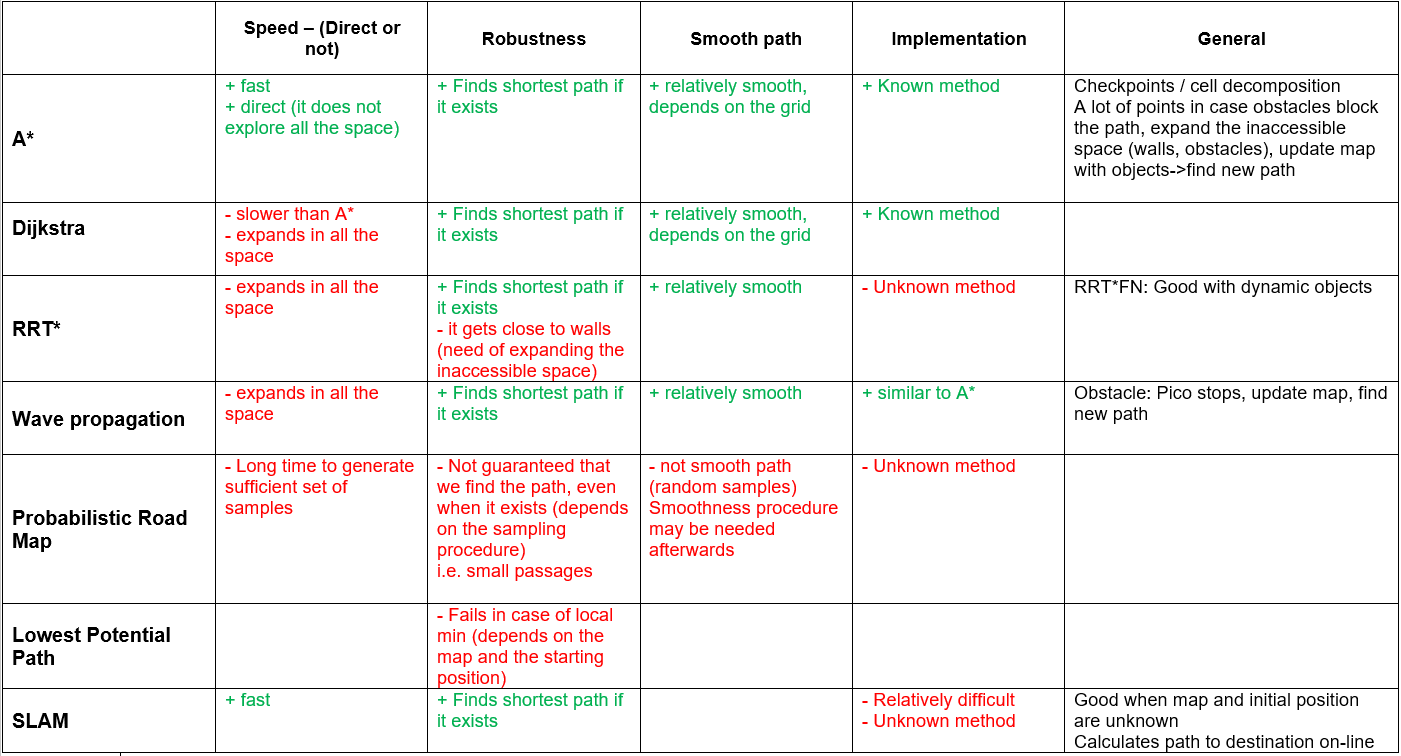
For the selection of the algorithm used for pathplanning a table of pros and cons has been formulated, which includes the computer performance index. A* is given an index number of 100. The walls and Dijkstra compute indices are an educated guess. The others are based on the amount of nodes used in a study

Algorithm	Compute index
A*	100
Rapidly-exploring random tree	100
Potential field algorithm	40
Dijkstra	100 or higher
Wall following	100000
Wave propagation	100 or higher

In addition, several algorithms are compared with respect to the convergence speed, the robustness of the method, the smoothness of the resulted path, the difficulty of the implementation and the sufficiency for the hospital challenge. The results are demonstrated in the table .........

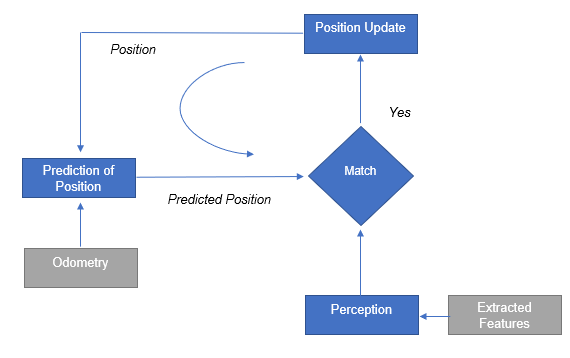
Localization

For the successful navigation it is important that the robot is aware of its current location. After considering known algorithms such as Markov localization, Kalman filter and partilce filters, it has been chosen to implement the line segment based localization. (Why?? restricted in time, experience, identification of landmarkes is problematic and etc.)

First, the algorithm requires features extraction and the most important features in the robot's environment are the straight lines. These features are used to build a local map from laser data. The line detection problem consists of two subproblems, namely segmentation, which deals with the number of lines and correspondence of data points to lines, and line extraction, which answers how to estimate line parameters, given which points belong to which lines. To solve the line extraction problem, we applied least squares solution. For the segmentation, the split and merge algorithm is used, which is a recursive procedure of fitting and splitting.

Split and Merge Algorithm:
Initialize set S to contain all points.
Split
* Fit a line to points in current set S.
* Find the most distant point to the line.
* If distance > threshold, then split and repeat with left and right point sets.
Merge
* If two consecutive segments are close/collinear enough, obtain the common line and find the most distant point.
* If distance <= threshold, merge both segments.

Logs

Meeting #	Date and Location	Agenda	Meeting notes
1	Date: 28-04-20 Time: 10:00 Platform: MS Teams	Introduction meeting with the tutor Discuss assignments to be completed Discuss preliminary design and algorithm	The tutor's role is to guide and answer questions. The tutor is present at the weekly meetings. Robot description: the unit of the laserdata distance is [m] and the angle is in radians; the robot is about 40*20cm. Gitlab should be used. Wiki: At the end of every meeting a clear list has to be made of actions that should be done. Important dates and information about the first assignment: The design document should be hanged in on may 4th as PDF and text should not be mentioned on the wiki. Design document is the document that describes how the software will look like, which includes requirements, functions, components, specifications and interfaces. Requirements: robot's speed constraints and etc.
2	Date: 01-05-20 Time: 14:00 Platform: MS Teams	Discuss the progress Discuss the design document	"Specifications and Requirments" section of the document design was discussed Finite State Machine was reviewed Action: meet on Monday (4th of May) to proof-read the report
3	Date: 05-05-20 Time: 10:00 Platform: MS Teams	Questions about odometry data Question on identifying exit Questions on GitHub Discuss work to be done	The robot overshoots on rotating to the right. This is much worse than when rotating to the left. The tutor is going to look into the simulator since it might be a bug in there. He will let us know as soon as possible. Take into account that the exit could be aligned with the sidewall of the room meaning there is only one corner to identify instead of 2. Gitlab tutorial is online and should help us figure it out. Group members have to use git clone to get the master repository on their pc. Slip is not enabled by default. It has to be enabled in the config file when needed in simulation. Work division Wall following algorithm -> Bjorn and Marzhan Wall finding and exit scanning -> Spyros and Arjun Wall alignment and exit corridor movement -> Bart and Joey
4	Date: 11-05-20 Time: 11:00 Platform: MS Teams	Discuss problems of code. Question on exit width.	good progress has been made on the initial scanning Joey and Bart had the same section of code. The alligning at the exit can be improved Work division Exit allignment -> Bjorn and Marzhan and Bart Wall finding and exit scanning -> Spyros and Arjun and Joey
5	Date: 18-05-20 Time: 11:00 Platform: MS Teams	Discuss what went wrong in the Escape room competition. Discuss Hospital Challenge.	Code is corrected and escape room is succeeded Brainstorm on hospital challenge Outlining main parts of the hospital challenge Work division Localization -> Bjorn and Joey and Marzhan Path Planning-> Spyros and Arjun and Bart
6	Date: 22-05-20 Time: 14:00 Platform: MS Teams	Discuss algorithm proposed by each subgroup. Discuss possible extension to object detection.	For path planning: A* For localization: particle filter Structure of the algorithm is constructed: main parts such as localization, path planning and object detection communicate through WorldModel and path planning also sends data to driveControl Work division is the same Localization -> Bjorn and Joey and Marzhan Path Planning-> Spyros and Arjun and Bart
7	Date: 26-05-20 Time: 11:30 Platform: MS Teams	Discuss progress in path planning and localization.	For path planning, A* algorithm: advantage of diagonal over Euclidian heuristic or vice versa. For localization: split and merge implementation for features extraction. Run localization all the time Work division is the same Localization -> Bjorn and Joey and Marzhan Path Planning-> Spyros and Arjun and Bart
8	5	10	15