Group2 19-1 Week5: Difference between revisions
No edit summary |
No edit summary |
||
| (9 intermediate revisions by the same user not shown) | |||
| Line 57: | Line 57: | ||
{| | {| | ||
|- | |- | ||
! | ! | ||
! scope="col" width="width:20em;" |Volume | ! scope="col" width="width:20em;" |Volume | ||
! scope="col" width="width:20em;" |Weight | ! scope="col" width="width:20em;" |Weight | ||
| Line 66: | Line 66: | ||
|- | |- | ||
|Propulsion | |Propulsion | ||
|~10x10x5 cm | |||
|3 kg | |||
|- | |- | ||
|*Up-down | |*Up-down | ||
| Line 108: | Line 110: | ||
|new prototype battery has power density of 10 microwatts per cubic centimeter, so for 100 W size of 10 m<sup>3</sup>? | |new prototype battery has power density of 10 microwatts per cubic centimeter, so for 100 W size of 10 m<sup>3</sup>? | ||
|- | |- | ||
| | |Communications | ||
|~10x5x5 cm | |~10x5x5 cm | ||
|~200 g | |~200 g | ||
| Line 134: | Line 136: | ||
In this case it is important to consider the balance between influx and outflux of energy. The digger needs to heat the ice in front of it enough to melt and possibly vaporise it, and the ice around it to allow the water to pass it by. At the same time, the surrounding ice tries to take away energy, preventing the water from freezing. | In this case it is important to consider the balance between influx and outflux of energy. The digger needs to heat the ice in front of it enough to melt and possibly vaporise it, and the ice around it to allow the water to pass it by. At the same time, the surrounding ice tries to take away energy, preventing the water from freezing. | ||
Because QuickField cannot model phase changes, we will be approximating the phase changes by a temperature difference. | Because QuickField cannot model phase changes, we will be approximating the phase changes by a temperature difference. | ||
The latent heat of fusion of ice is 334 kJ/kg. At 0 degrees Celcius, ice has a specific heat of 2.050 kJ/kg K. Dividing l/ | The latent heat of fusion of ice is 334 kJ/kg. At 0 degrees Celcius, ice has a specific heat of 2.050 kJ/kg K. Dividing l/C<sub>v</sub> gives ΔT=334/2.050=163 K. [https://www.engineeringtoolbox.com/latent-heat-melting-solids-d_96.html Latent heat of fusion solids] | ||
[https://www.engineeringtoolbox.com/ice-thermal-properties-d_576.html Ice specific heats] | [https://www.engineeringtoolbox.com/ice-thermal-properties-d_576.html Ice specific heats] | ||
That means that in the model, temperatures between 0 and 163 degrees Celcius correspond to a phase change between ice and water. Below 0 degrees is pure ice of temperature T, and above 163 degrees is pure water of temperature T-163. | That means that in the model, temperatures between 0 and 163 degrees Celcius correspond to a phase change between ice and water. Below 0 degrees is pure ice of temperature T, and above 163 degrees is pure water of temperature T-163. | ||
Three pictures made are shown below: | Three pictures made are shown below: | ||
The pictures display a source in the front of the digger, with a total output of (from top to bottom) 1, 4 and 10 kW. | |||
Furthermore, the isotherms in the picture are spaced by 163 degrees Celsius, starting at -163 degrees. That means that the outermost isotherm indicates still far below freezing temperatures, but the 2nd and 3rd outermost isotherms indicate the lower and upper edges of the freezing regime. | |||
[[File:front_source_1_kw.jpg|thumb|upright=1.0|right|Fuel source of 1 kW in the front]] | [[File:front_source_1_kw.jpg|thumb|upright=1.0|right|Fuel source of 1 kW in the front]] | ||
This shows that at 1 kW, the digger is unable to melt any of the surrounding ice. At 4 kW it is visible that a small layer just in front of the digger will melt completely. | |||
[[File:front_source_4_kw.jpg|thumb|upright=1.0|right|Fuel source of 4 kW in the front]] | [[File:front_source_4_kw.jpg|thumb|upright=1.0|right|Fuel source of 4 kW in the front]] | ||
However, as the digger would progress and this water would flow to the back of the digger, it will quickly return to the half-molten regime. | |||
It is only at 10 kW that we see the digger form a significant layer of fully molten water around it, with a thickness of between 10 and 20 cm. | |||
[[File:front_source_10_kw.jpg|thumb|upright=1.0|right|Fuel source of 10 kW in the front]] | [[File:front_source_10_kw.jpg|thumb|upright=1.0|right|Fuel source of 10 kW in the front]] | ||
However, not even halfway down the length of the digger, all this water will refreeze. | |||
As shown in the next picture, to warm the entire digger 100 kW is required. | |||
This is obviously a lot, and based on the model a large portion of the equipment would reach absurd temperatures. Furthermore, in the model, convective processes are neglected so the actual necessary energy could even be a factor of order 1 larger or smaller. | |||
[[File:front_source_100_kw.png|thumb|upright=1.0|right|Fuel source of 100 kW in the front]] | [[File:front_source_100_kw.png|thumb|upright=1.0|right|Fuel source of 100 kW in the front]] | ||
To overcome this, a very small, long heat source along the length of the digger is proposed. In this case, the same water layer can be established, but with only 20% the total power: 10 kW for both sources. Mind you that this power source is a hollow cylinder. | |||
[[File:front_source_side_channel.png|thumb|upright=1.0|right|Fuel source in the front with two side channels]] | [[File:front_source_side_channel.png|thumb|upright=1.0|right|Fuel source in the front with two side channels]] | ||
[[File:front_source_rings_4_kw.png|thumb|upright=1.0|right|Fuel source of 1 kW in the front]] | The last configuration is one in which the cylinder has been replaced by two rings that produce 4 kW each. | ||
[[File:front_source_rings_4_kw.png|thumb|upright=1.0|right|Fuel source divided into two rings of 4 kW]] | |||
Furthermore, from the power output of the source, the maximum speed of the digger can be determined. This is based on the amount of ice in front of the digger that it can melt per second. | |||
The energy H required per kilogram of ice to melt it is equal to: | |||
''H = l<sub>f</sub>+C<sub>v</sub>*ΔT'', where ''l<sub>f</sub>'' is the latent heat of fusion, the energy required to induce a phase change, ''C<sub>v</sub>'' the heat capacity and ''ΔT'' the temperature difference to be established. for ice, ''l<sub>f</sub>''=334kJ/kg, ''C<sub>v</sub>''=2.05kJ/kg/K and in the case of Europa ''ΔT''=50 -- 200 = 250K at least. The lower temperature boundary is the lowest temperature we expect to encounter on Europa, whereas the upper temperature boundary is there to ensure that the water stays molten for a while. This temperature difference will likely decrease as the digger gets deeper into the crust, because the ice warms up slowly. However, in the worst case this will allow the digger to go somewhat faster. | |||
The total energy then becomes H=334+2.05*250=846.5kJ/kg. The energy per unit volume can be determined from the density of ice: 920 kg/m<sup>3</sup>, resulting in 778780 kJ/m<sup>3</sup>. | |||
The digger’s speed v can be calculated by dividing the total power used to melt the ice directly in front of it P by the energy required per unit volume H and the total frontal area of the digger A. The latter is equal to π*r<sup>2</sup>, where r is the radius of the digger's cylindrical part. ''v = p/(H*π*r<sup>2</sup>)'' | |||
For instance, at 10 kW and a radius of 25 cm, the velocity of the digger becomes 65 μm/s. At this velocity, the digger would take about 4.8 years to dig 10 km. At 1 MW effective power, this is reduced to a much more manageable 17.7 days. | |||
'''Scaling up the power source''' | |||
Considering the amount of power required for the digger, accounting for losses is a luxury not available to us. Hence, the heat production should be done as close to the place where it is needed, namely in front of the digger. An additional advantage of this is that it will weigh the digger down, meaning it may push itself through the ice a little faster. | |||
[https://web.archive.org/web/20121218194925/https://netfiles.uiuc.edu/mragheb/www/NPRE%20402%20ME%20405%20Nuclear%20Power%20Engineering/Radioisotopes%20Power%20Production.pdf RTG source selection] (If not mentioned otherwise, radioisotope data in the remainder of this section is derived from this document.) | |||
There are several consideration to be made when selecting a power source for an RTG: | |||
*Sufficient (power) density | |||
*Sufficient duration | |||
*Right type of radiation | |||
*Non-critical | |||
'''(Power) density''' | |||
In particular in space missions, the power density of the source is important. For instance, it is possible to get a block of Am<sup>241</sup>O<sub>2</sub> that produces 10 kW, but at a power density of .097 thermal Watts per gram, this block would weigh 103 kg. As a comparison, Am<sup>244</sup><sub>2</sub>O<sub>2</sub> has a power density of 2.01 Wh/g, resulting in 10 kW of power at only 4.98 kg. | |||
Furthermore, the material's density itself matters as well. To illustrate: Cs<sup>137</sup>Cl has a power density similar to Am<sup>241</sup>O<sub>2</sub>, but not 1/3 the density. As a result, a block of Cesium Chloride must be 3 times as large to produce the same power as a block of Americium Dioxide, whereas both blocks have about the same mass. | |||
Thus, the perfect power source should have a large enough power density to decrease its mass, as well as a high enough mass density to decrease its volume. | |||
The mass of the power source is equal to the desired power divided by the power density. This mass should be divided by the mass density to find the volume of the power source. | |||
'''Duration''' | |||
One disadvantage of RTGs is that due to the decay of the radioactive source, the power of the source slowly decreases over time. An isotope with a sufficient half-life should thus be chosen accordingly. | |||
Consider 3 isotopes: | |||
*Uranium-235, with a half-life of 8.9*10^8 years [https://pubs-rsc-org.dianus.libr.tue.nl/en/content/articlepdf/1953/an/an9537800394 Uranium half-life] | |||
*Plutonium-238, with a half-life of 87.74 years | |||
*Cerium-144, half-life of 284.4 days. | |||
The activity of isotopes is proportional to the number of isotopes. In particular: | |||
''A(t) = A(0)*2<sup>(-t/t<sub>(1/2)</sub>)</sup>'' with ''A(0)'' the initial activity (to which power production is proportional) and ''t<sub>(1/2)</sub>'' the half-life of the radioactive source. | |||
Filling in the half-lives for ''t = 7'' years, a rough estimate of the travel time to Europa, results in activities at the time of arrival of 1, 0.946 and 0.00198 times the initial activity for U-235, Pu-238 and Ce-144 respectively. This goes to show that an isotope like Cesium-144 would reduce in power by 99.8% over the course of the journey to Europa. To achieve a power of 10 kW upon arrival, the initial power source must then be 5 MW. This power requires a 200 kg Ce source. Uranium and Plutonium, on the other hand, retain most of their activity over the course of the mission. | |||
However, this would imply that Uranium would be a better source than Plutonium. This is not the case. Even though the activity of U decreases more slowly than Pu, the activity of Pu is higher than U. This can be understood by realising that U has a longer half-life ‘’because’’ it decays slowly. If it decayed faster, all the atoms would deplete in a much shorter timespan. There is a general inverse correlation between activity and half-life. As a result, the half-life of the perfect isotope is too high nor too low, preferably about an order of magnitude greater than the duration of the mission. | |||
The power density upon leaving earth can be calculated as follows: | |||
''P(t) = P(0)*2<sup>(-t/t<sub>(1/2)</sub>)</sup>''. | |||
'''Type of radiation''' | |||
Now we shall consider the type of radioactive decay that the perfect radioactive source undergoes. | |||
To avoid radioactive contamination of environments or the destruction of on-board equipment, it is important to shield the radioactive source. At the same time, due to weight considerations, it is also important that said shielding is not too thick. | |||
To achieve the latter, the right type of radiation has to be chosen. Of the four main types of decay - alpha, beta, gamma and neutron - alpha is by far the most easy to mitigate, with air half-value layers of the order of cms. Hence, it also makes sense to choose a radioactive source that decays via alpha radiation. Examples are Plutonium-238 and Curium-244. | |||
'''Non-critical''' | |||
Lastly it is important that the mass of the radioactive isotope does not exceed its critical mass. If a hunk of the isotope exceeds the critical mass, the decay of atoms in the isotope will accelerate the decay of other atoms in the isotope, causing a runaway chain reaction like the ones that occurred in Tsjernobyl and Fukushima, like the ones that are induced in nuclear bombs. If the mass is kept small enough, or the geometry is chosen efficiently, the atoms don’t interact with each other as much and the chain reaction will remain under control. | |||
These comparisons hopefully suffice to illustrate why a runaway chain reaction is undesirable. | |||
The considerations to be made are as follows: | |||
*’’High critical mass of the isotope’’: This allows for a larger amount of isotope to be taken along on the mission. | |||
*’’Geometry’’: As in a spherical configuration the atoms are most densely packed together, the critical mass is lower in a sphere. [https://inis.iaea.org/collection/NCLCollectionStore/_Public/07/244/7244852.pdf?r=1&r=1 Critical masses] | |||
'''The best power source''' | |||
Below is presented an overview of the most promising radioactive sources, with from left to right their half-life, power density, mass density, critical mass, P0, mass, volume, attenuation layer. To determine what source is ‘most promising’, the right principal type of radiation and the right half life of some isotopes were picked from the article on RTG source selection mentioned in the beginning of this section. Most information was gathered from that article as well. | |||
To determine the attenuation layer, the lead layer thickness required to reduce the gamma dose (alpha does not transmit through lead at all) at 1 m to .1 mSv. | |||
In this article ([https://www.osti.gov/servlets/purl/4827212 Curium attenuation]), the shield required to meet precisely that condition is given for Curium with lead. For other sources this information appears impossible to find. Thus, to get an order of magnitude estimation of the other materials, we will be using the ratio between this value and the values listed in the article on RTG power sources. With an attenuation thickness for Cm-244, this ratio is 2.155. Results are in the table. | |||
[https://www.tandfonline.com/doi/pdf/10.1080/18811248.2002.9715296 Curium mc] | |||
[https://www.tandfonline.com/doi/pdf/10.13182/NSE07-A2644?needAccess=true Plutonium mc] | |||
[https://inis.iaea.org/collection/NCLCollectionStore/_Public/36/116/36116528.pdf?r=1&r=1 Americium mc and density] | |||
The isotopes chosen were Curium-244, Plutonium-238 and Americium-241. | |||
{| border="1" cellpadding="2" | |||
|---- | |||
! | |||
! scope="col" width="width:20em;" |t(1/2) (a) | |||
! scope="col" width="width:20em;" |ρ (kg/L) | |||
! scope="col" width="width:20em;" |ρw (W/kg) | |||
! scope="col" width="width:20em;" |mc (kg) | |||
! scope="col" width="width:20em;" |P(0) (W) | |||
! scope="col" width="width:20em;" |m (kg) | |||
! scope="col" width="width:20em;" |V (L) | |||
! scope="col" width="width:20em;" |r (dm) | |||
! scope="col" width="width:20em;" |d (dm) | |||
! scope="col" width="width:20em;" |ml (kg) | |||
|- | |||
|Cm | |||
|18.11 | |||
|13.51 | |||
|2840 | |||
|20.07 | |||
|13072 | |||
|4.6 | |||
|0.341 | |||
|.433 | |||
|1.1 | |||
|167.4 | |||
|- | |||
|Pu | |||
|87.74 | |||
|19.33 | |||
|560 | |||
|8.2 | |||
|10568 | |||
|18.9 | |||
|0.976 | |||
|.615 | |||
|0.0547 | |||
|3.224 | |||
|- | |||
|Am | |||
|432.0 | |||
|13.66 | |||
|110 | |||
|56.4 | |||
|10113 | |||
|91.93 | |||
|6.73 | |||
|1.17 | |||
|.3832 | |||
|102.1 | |||
|---- | |||
|} | |||
The two most important conclusions to be drawn from the table are the following: | |||
Cm, at the required mass, has less than a quarter of its critical mass. Pu and Am have approximately 2 times the mass. So, Cm can be used as a solid sphere, whereas for Pu and Am, a different geometry would have to be chosen, or multiple smaller spheres have to be used. | |||
Furthermore, disregarding the critical masses of the isotopes, Cm would require a sphere with a radius of 15.3 cm, weighing 172 kg; Pu a sphere of radius 6.7 cm, weighing 22.1 kg; and Am radius 15.5 cm, weighing 194 kg. | |||
Considering the critical and required mass of Americium, it can be quickly dismissed as a useful source. | |||
Thus, to choose between either Cm or Pu, we will attempt to make different suggestions for the geometry of the Plutonium, and determine whether the different geometries still result in lower masses than Curium. | |||
The first is to split the single plutonium core into 3 separate cores. The mass of these will be the same, but each will now have a radius of 4.27 cm. To coat these in a layer of .547 cm of lead, requires 1.6 kg each, or a total of 4.8 kg, bringing the total mass up to 23.7 kg. | |||
However, this configuration does not lend itself for a very homogeneous temperature distribution. For that, we will use a hollow hemisphere with a thickness of half the critical radius (rc=4.66 cm). To obtain the required mass of 18.9 kg, this sphere should have an inner radius of 6.97 cm. To coat this hemisphere with the required layer on both in- and outside (the outside for protection of the environment, and the inside for protection against itself), requires 10.0 kg of lead, for a total of 28.9 kg. | |||
The last suggested configuration is a torus with a square cross section with a diagonal cross section equal to the critical radius as listed [https://inis.iaea.org/collection/NCLCollectionStore/_Public/07/244/7244852.pdf?r=1&r=1 here] (4.04 cm). The major radius of this torus is then 9.52 cm. To cover this torus in lead, requires 6.8 kg of lead, bringing the total mass to 25.7 kg. | |||
Each of these configurations has its own benefits and disbenefits. The 3 spheres probably don’t create a very homogeneous temperature distribution, which may cause the digger to lose efficiency when digging, or have trouble going straight. The hollow sphere is the heaviest of the 3 configurations, and the torus fails to heat the front of the digger, where the heat is needed most. | |||
The best solution can be found in a combination. The idea is to create a sphere of plutonium with a torus around it. The sphere and torus are separated by a double layer of lead, then the radii are varied to get the exact right mass of plutonium. The cross section of the configuration is shown in the figure below: | |||
Calculations give a radius of the sphere of 4.47 cm, still below the critical radius, and a major radius of the torus of 7.04 cm. | |||
The total mass of shielding is 6.4 kg, resulting in a total of 25.3 kg. This is still a bit above the mass of the spheres, but a little below the mass of the single torus. The configuration is radially symmetric, and due to the smaller torus radius can be placed higher up in the digger. | |||
[[File:plutonium_sphere_torus.png|thumb|upright=1.0|right|Construction of the plutonium sphere and torus]] Furthermore, if deemed necessary for sufficient heat transfer, the sphere can be pushed up a little further than the torus as well. This way the Plutonium-238 does not reach critical masses, and the total mass is still well below the mass of the total Curium-244 source. | |||
In conclusion, the best power source for the digger is Plutonium-238, due to its high alpha ray energy density. The configuration of source material best suited for our purposes is the sphere-torus combination. | |||
'''The design of the spacecraft''' | |||
The lander that we envision consists of three different parts that, at first, land on the planet as one vehicle. This lander then splits off a digger vehicle (not sure where this digger vehicle will be on the lander yet), which makes its way through the solid ice. The lander itself will remain on the surface, whilst physically remaining connected to the digger. This physical connection consists of a wire (fiberglass or electrical) that runs between the digger and the lander. This physical link was chosen because of the ice on Europa. This ice will most likely freeze shut any tunnel that the digger makes, which makes a wireless connection through the ice extremely unusable. This lander is furthermore equipped with a few tools to perform some experiments on the surface. These tools consist mainly of the same tools that the digger has on board, namely a seismometer, a spectrometer, a camera, and a tool to perform gas emission experiments. | |||
When the digger reaches the underside of the ice and is about to enter the water, the digger locks itself into the bottom of the ice. Inside this digger is a compartment that houses an AUV. This compartment will open to let the AUV into the water. The digger itself will remain stationary in the ice and communicate through sound waves with the AUV. The AUV, then, will start exploring the subsurface ocean as a submarine, if you will. During this exploration, this submarine will take pictures and perform spectrometric experiments and gas emission experiments. | |||
'''Communication between the lander and Earth''' | |||
On Earth, NASA uses a system called DSN to communicate with spacecraft [https://deepspace.jpl.nasa.gov/about/ NASA DSN]. DSN stands for Deep Space Network, and it allows NASA to communicate with spacecraft as far as Opportunity 1, which is, by now, outside of our solar system. This means that a satellite around Europa is not absolutely necessary, but it may provide a faster way to get our data to Earth. Since Europa is tidally locked to Jupiter ([https://phys.org/news/2015-09-jupiter-moon-europa.html as written here]), and since its orbital period is about 3.5 earth days ([https://solarsystem.nasa.gov/moons/jupiter-moons/europa/in-depth/#orbit_and_rotation_otp as stated here]), a large part of the time, the lander will not be able to directly send data back to Earth. This is due to either Jupiter being in the way, or Europa itself is in the way when the lander is at the back of Europa, relative to Earth. If, for this mission, there was another satellite orbiting Europa or Jupiter, the lander would be able to send its data to this satellite with a much higher data rate. During the Mars Curiosity mission, NASA has an orbiter around Mars, which intermittently communicates with the Rover. The communications between Curiosity and this orbiter are about 150 times as fast as the communications would be between Curiosity and Earth directly. Since this orbiter has more power that it can focus on communications, the data will reach Earth much faster through this orbiter, than it would directly to Earth.[https://mars.nasa.gov/msl/mission/communications/#data Communications of Curiosity]. | |||
'''Necessary bandwidth''' | |||
Of all the instruments that our Europa lander is expected to carry, the camera is expected to generate the most data. During the Mars Exploration Program, which has already lasted for over a decade, the camera of Opportunity would generate an expected 97.36 Gigabytes of data ([https://pds-geosciences.wustl.edu/missions/mep/datarecomm.pdf as budgeted here]). Since our mission is not expected to last nearly as long, the expected bandwidth necessary for this mission is also not expected to be particularly large. If our mission is expected to only last for a fraction of the time that the Mars Exploration Program has already lasted, an orbiter may not even be necessary to obtain all data generated on Europa within reasonable time. | |||
'''Wireless communication through ice''' | |||
Ice is quite a good isolation material when it comes to wireless signals. Radio signals are very quickly absorbed by ice ([https://agupubs.onlinelibrary.wiley.com/doi/full/10.1029/2005JB003903 this study found]), and optical signals do not travel through ice very far either. Low frequency radio waves, however, are a medium that does travel through ice better ([https://www.era-learn.eu/network-information/networks/eurostars/eurostars-cut-off-2/through-ice-location-and-communication-system as written here]). The question arises, however, whether these radio waves will survive through 10 km of ice, as that would be a very unusual situation here on Earth for researchers to test on. | |||
So we assume wireless communication through the ice crust on Europa is for all intents and purposes impossible. | |||
'''Electrical cable as alternative to fiberglass''' | |||
In current society, most electrical cables are made of copper or aluminum. These cables are used for their high conductivity and low resistance. Where aluminum is mostly used for heavy industrial wirings, copper is at the core of most everyday electrical cables. Copper’s conductivity decreases as temperature increases, but as the temperature goes down, the conductivity increases. This happens until about 30 Kelvin,at which point lower temperatures drastically decrease copper’s conductivity. Even though copper’s conductivity at around 30 Kelvin reaches a very high point, at no point is copper itself considered to be a superconductor. | |||
[[File:thermal_conductivity_copper.png|thumb|upright=1.0|right|graph of copper conductivity vs temperature]] | |||
[https://www.researchgate.net/figure/Variation-of-thermal-conductivities-of-copper-and-stainless-steel-with-temperature_fig4_319575524 Source] | |||
'''Where will the experiments be performed?''' | |||
Both the initial lander, as well as the Autonomous Underwater Vehicle (AUV) possess instruments that will be used to perform experiments. The initial lander features one more instrument than the AUV does, and the lander will remain stationary on the surface. Here, the lander will perform the spectrometric experiments on the ice of the surface, as well as gas emission experiments. Furthermore, the lander will deploy its seismic sensor on the ice to perform seismic readings, as well as use its camera to take pictures of its surroundings. | |||
The AUV will make its way into the subsurface ocean in order to make valuable use of its sensors. Save for the seismic sensor, the AUV is equipped with the same tools as the lander. The AUV will perform spectrometric observations on samples of water, as well as any soil, if there is anything of the like present. The AUV will also perform gas emission experiments on samples of the same material (e.g. water at different depths and soil, if available). Since it wouldn’t make sense for the AUV to perform seismic experiments in the water, the only other thing that the AUV will be doing is taking photos from underwater (and hopefully capture some life on them). | |||
'''Movement under water''' | |||
To be able to manoeuver under water, the AUV needs to have a propulsion system. The simplest propulsion system that would (probably) work, is one (or more) propellers, like the ones that submarines have. Underwater drones are already available to the public, and most of them seem to feature this style of propulsion. | |||
Another alternative propulsion method may be one that is designed by means of biomimicry. One example of such a project can be seen to the right ([https://www.noa-marine.com/ NOA Marine]). In this image, the drone mimics the movements of a squid to propel itself forth. Besides this design, there have been multiple other designs that rely on biomimicry, for example mimicking a manta ray. | |||
[[File:prototype_AUV.png|thumb|upright=1.0|right|prototype of biomimetic propulsion system]] | |||
''Sluiswerking op extreme dieptes is helaas niks over te vinden'' | |||
'''What to do with extraterrestrial life (updated)?''' | |||
It is, of course, not impossible that a mission to Europa will find life of some form or another. We will then wish to learn from this life, but the ways in which we can ethically allow ourselves to do so may be limited. The question is: How limited? Should we find extraterrestrial life, how does this impact the mission? | |||
To answer this question, it is important to first consider the moral value of extraterrestrial life in itself. | |||
The following dissertation outlines why all life, terrestrial or not, should be considered to have some intrinsic value. We adopt this view, acknowledging that at the same time extraterrestrial life has extrinsic value as well, as it can serve to further scientific research ([https://www.cambridge.org/core/journals/international-journal-of-astrobiology/article/does-extraterrestrial-life-have-intrinsic-value-an-exploration-in-responsibility-ethics/5DCA161726CE8F4FC9E58EE8E6D04B81 as argued in this article]). | |||
Furthermore, one should note that, if something does not have intrinsic value, this does not mean it should not be preserved. If something has value to something with moral value, it acquires value. So it also goes for the Europan environment, which is important for the life present on it, as well as for our own research purposes. | |||
We will discuss some different forms of life that we might encounter during the mission and how we should handle in each individual case. | |||
First of all, sentient versus non-sentient life. If we find life at all, it is far more likely that it will be microbes or other non-intelligent alien life than sentient otherworldly beings ([http://blogs.discovermagazine.com/d-brief/2018/02/16/react-discovery-of-alien-life/#.XYy4iVUzapo as argued here]). As stated in previous paragraph, all life should be considered to possess intrinsic value, which means that we would treat the potential sentient and non-sentient life the same way. In the (previous/following) section experiments, one can read the experiments that we would like to examine on Europa. '''None of the experiments will harm or disturb the potential life directly.''' But the life can be harmed indirectly too, which we will explain later on this section. | |||
Second of all, carbon-based life versus non carbon based life. On earth, all known living things are based on the element carbon. But scientists belief that there could be an alternative chemical basis for life, for example silicon-based life ([https://link-springer-com.dianus.libr.tue.nl/content/pdf/10.1007%2Fs12633-014-9254-7.pdf according to this article]). There could be creatures in unimaginable forms and capabilities on Europa, but since it is also “life” we will treat these potential creatures the same as carbon-based life, with the assumption that they have some sort of intrinsic value. | |||
Another thing that needs to be excluded, is the possibility of contamination and thereby affecting Europa’s environment or ecosystem. This is the indirect harm of life on Europa as addressed before. Contamination can be done in different ways; via human bacteria or viruses that end up on the robot during the construction of it, wear or rust of the metals in the robot or a radioactive source that poisons the environment. | |||
The first possible way of contamination, human bacteria, seems to be no problem at all. [https://www.quora.com/Does-freezing-kill-bacteria-How-cold-does-it-have-to-be According to the following source], bacteria die slowly when they are exposed to freezing temperatures. And since the robot is traveling through the space for about 6 years in -270 degrees of Celsius, we can assume that all possible human bacteria are dead when the robot arrives on Europa. | |||
Viruses are a higher risk for contamination. There are multiple researches done to the surviving of viruses in spatially conditions, and some experiments proved that viruses can actually survive in these conditions for a long time. The longest time a virus was exposed to (and survived) the space environment was 2 years. Since there is no data of the decline of this specific virus, we can not make a prediction if this virus will survive the 6 travel years to Europa. And thereby we can not exclude that some viruses might be transferred from earth to Europa during the mission. The only thing we can do is make sure that the robot goes into space as virus-free as possible ([https://www-liebertpub-com.dianus.libr.tue.nl/doi/10.1089/ast.2017.1649 as can be read here]). There is also a possibility that the robot could catch a virus on its way to Europa. But to date, almost no research has looked into the possibility of viruses “living” in space. So until this remains unknown, we don’t know if we have to take [http://www.astronomy.com/news/2018/01/the-hunt-for-viruses-in-space this risk into account]. | |||
For the last two ways of contamination, wear or rust of the metals in the robot or a radioactive source that poisons the environment, the choice for materials and energy source should take these dangers into account. So the materials should be the best non-wearing and non-rusting materials available. And we must look for an energy source that does not have radioactive leakage, so the environment of Europa won’t be in danger. '''We will discuss this later on.''' | |||
'''Structure''' | |||
*Introduction - general knowledge about Europa (week 1) | |||
**Physical consideration about the presence of water on Europa (week 4) | |||
**Presence of a sub-surface ocean (week 4) | |||
**Indications for life | |||
*Problem statement (partly week 1) | |||
*Users (week 3) | |||
**User requirements (week 4) | |||
**User preferences (week 4) | |||
**Constraints (week 4) | |||
**Measurability requirements and constraints | |||
*On the ice or in the water? Examine Europan core - section 3.2 (week 3) | |||
*Experiments | |||
**Which experiments (week 4) | |||
**Where to execute (week 5) | |||
*General | |||
**Communication | |||
***Earth to surface receiver (partly week 5) | |||
****Via satellite? | |||
****Necessary bandwidth | |||
***Surface receiver to sub-surface receiver (week 4) | |||
****Fiber options | |||
****Electric cable options (week 5) | |||
****Wireless communications through ice | |||
***Communications under water (week 4) | |||
****Wireless communication | |||
**Autonomous | |||
** What to do with extraterrestrial life (week 5) | |||
***Sentient vs non-sentient life | |||
***Carbon-based vs non-carbon-based life | |||
***Possibility of contamination | |||
**How long does the mission need to last (week 5) | |||
*From Earth to Europa | |||
**General description about the journey and the risks | |||
*Landing and surviving on the surface | |||
**Where to land (week 4) | |||
**Tectonic activity (week 2) | |||
**The effect of temperature and pressure on the functioning of the robot (week 2) | |||
**Shape and function of the lander | |||
**Executing experiments | |||
*Through the ice - from surface to the sub-surface ocean | |||
**Power | |||
***Power source in digger or on surface (week 5) | |||
***Estimating how much power is needed (week 5) | |||
***The most suited power source (week 5) | |||
**Functioning of an RTG on Europa (week 2) | |||
**Where to place a sub-crust base (week 4) | |||
**Modelling temperature on the digger (week 4) | |||
** Shape and function of the digger and sub-crust base | |||
**Executing the experiments | |||
*In the water | |||
**Movement submarine (week 5) | |||
**Is it possible to go to the seafloor (week 4) | |||
**Shape and function of the submarine (partly week 5) | |||
**Executing the experiments | |||
*Design (partly week 5) | |||
**Submarine | |||
**Sub-surface crust | |||
**Ice digger | |||
**Lander | |||
*Discussion | |||
*Planning | |||
[[PRE2019_1_Group2|Back to the main page]] | [[PRE2019_1_Group2|Back to the main page]] | ||
Latest revision as of 08:49, 7 October 2019
How long does the mission need to last?
Europa could possibly be regarded as being spherically symmetric, were it not for Jupiter’s strong gravitational influence on her. Many processes are probably highly influenced by Jupiter, either positively or negatively. Since we don’t know for sure what processes can sprout life and/or how those processes are influenced by external gravity, it can be interesting to search for life both on the Jovian and the Anti-Jovian side of Europa.
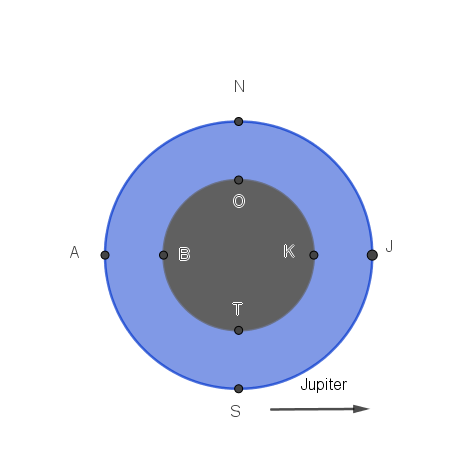
Regard the picture to the right. The surface points indicate the surface of the ocean, below the crust, and are noted as Jovian (J), Anti-Jovian (A), North (N) and South (S). The seafloor points have the same letters, shifted 1 forward. To search the Jovian and Anti-Jovian poles, the lines AB and JK make the most sense. Have the submarine sail across these lines and take a sample every so often. To travel between these lines, the semicircle BOK or BTK seems most logical because the radius of these circles is the smallest possible. The total route ATOKJ is 4700 km. However, that does require that the submarine can remain at seafloor levels of pressure for extended periods of time (on the order of years). To avoid this, the route ATANJK can be taken. This one, however, is 5320 km. The observant reader may have noticed that 5320 km is A LOT. A car driving on the Dutch highways will have to drive from Groningen to Maastricht and back almost 8 times. This will take roughly 60 hours. Bathyscaphe Trieste (the first submersible to reach the bottom of the Challenger Deep) submerged and resurfaced with an average speed of 0.9 m/s, with which it would complete the journey in 68 days, ignoring the fact that Trieste could only go up and down. The Trieste submersible had a mass of 14 metric tons. This is obviously much too big. However, it was made to harbor 2 people and protect them from the immense pressure, which we don’t need. The inside pressure needs only be small enough that the equipment can still function. On the other hand, our submersible needs to withstand double the pressure that Trieste did. Furthermore, Trieste used gravity to sink and rise, which is much lower on Europa. All in all, the maximum diving speed of the submersible may cap out well below 1/10 of Trieste’s speed. Furthermore, the lateral speed of the submersible is probably even lower, due to complicated driving systems. Since the exact velocity of the submarine is difficult to estimate precisely at this point, we will accept this 0.05 m/s as the actual top speed. At this velocity, the long route takes 3.37 years, and the short route ‘only’ 2.98 years. Only going back and forth between the ice and the seafloor takes 70 days. This is a total distance of 300 km. Please remember that this is calculated in a straight line, completely ignoring the fact that the route straight down may not be possible due to obstructions, or desirable due to interesting finds along the way. All this also disregards the dig down to the sea. Valkyrie achieved a velocity of 0.9 m/hr. This means it would take 46 days to dig through the crust. Combine this with a startup time, communications check, and pre-disembarking measurements, and the total time before setting off could easily be 60 days. Considering the interest in both the Jovian and anti-Jovian poles of Europa, the ATOKJ is likely most useful. To allow for deviations, we will add an estimated 41% length to the route; this will allow a deviation off the route of 45 degrees at all times. At an average speed of 0.05 m/s, this will take an estimated 1538 days, or 4.21 years. Add the 60 days to get 4.4 years. Plus some room for error, we’re looking at a mission of between 4.5 and 5 years.
Navigation
Going up and down by making the total density equal to water, but adding a piston filled with a fluid that is just slightly more compressible than water to the outside of the submarine.
Parts list
Surface base:
- Fixation stuff
- Power source
- Communications
- Emitter
- Receiver
- Research equipment
- Seismometer
- Spectrometer
- Camera
- Gas emission experiment tools
Digger:
- Digging stuff
- Power source
- Shielding
- Battery
- Accelerometer
- Fixation stuff
- Communications
- Emitter
- Receiver
- Cable
- The cable is with the digger to avoid having to drag all that length of cable all the way through the ice, and to make sure that it's possible to unroll the cable
Submarine:
| Volume | Weight | |
|---|---|---|
| Barometer | d=4 cm, r=5 cm | ~50 g |
| Propulsion | ~10x10x5 cm | 3 kg |
| *Up-down | ||
| *Sideways | ||
| Gyroscope | in computer | negligible |
| Accelerometer | in computer | negligible |
| Research equipment | ||
| *Gas emission | ~30x30x30 cm | ~2.5g |
| *Camera x2 (each) | 6.2x4.5x2.8 cm | 100 g |
| *Spectrometer | 6x8x2 cm | 100 g |
| Pressure-resistant hull | ~15 cm external | dependent on dimensions |
| Power source (100 W?) | 9 cm3 | 170 g |
| *Shielding | 0.51 cm | 200 g |
| *Reloadable battery | new prototype battery has power density of 10 microwatts per cubic centimeter, so for 100 W size of 10 m3? | |
| Communications | ~10x5x5 cm | ~200 g |
| *Emitter | ||
| *Receiver | ||
| Method to sample water | 1 L | 2 kg |
| Lights | 0.06 m3 | 1 kg |
| Computer | 1 L | 3 kg |
Model for digging through the ice
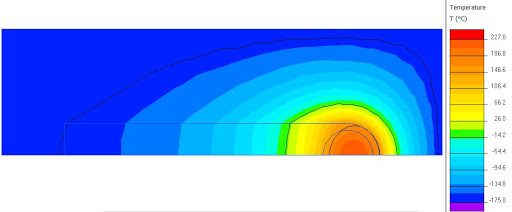
In this case it is important to consider the balance between influx and outflux of energy. The digger needs to heat the ice in front of it enough to melt and possibly vaporise it, and the ice around it to allow the water to pass it by. At the same time, the surrounding ice tries to take away energy, preventing the water from freezing. Because QuickField cannot model phase changes, we will be approximating the phase changes by a temperature difference. The latent heat of fusion of ice is 334 kJ/kg. At 0 degrees Celcius, ice has a specific heat of 2.050 kJ/kg K. Dividing l/Cv gives ΔT=334/2.050=163 K. Latent heat of fusion solids Ice specific heats That means that in the model, temperatures between 0 and 163 degrees Celcius correspond to a phase change between ice and water. Below 0 degrees is pure ice of temperature T, and above 163 degrees is pure water of temperature T-163. Three pictures made are shown below:
The pictures display a source in the front of the digger, with a total output of (from top to bottom) 1, 4 and 10 kW. Furthermore, the isotherms in the picture are spaced by 163 degrees Celsius, starting at -163 degrees. That means that the outermost isotherm indicates still far below freezing temperatures, but the 2nd and 3rd outermost isotherms indicate the lower and upper edges of the freezing regime.

This shows that at 1 kW, the digger is unable to melt any of the surrounding ice. At 4 kW it is visible that a small layer just in front of the digger will melt completely.
However, as the digger would progress and this water would flow to the back of the digger, it will quickly return to the half-molten regime. It is only at 10 kW that we see the digger form a significant layer of fully molten water around it, with a thickness of between 10 and 20 cm.
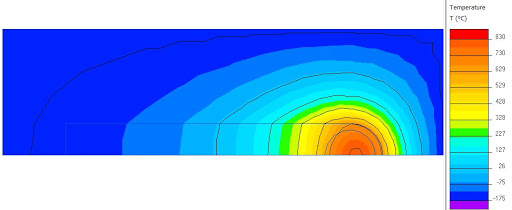
However, not even halfway down the length of the digger, all this water will refreeze. As shown in the next picture, to warm the entire digger 100 kW is required. This is obviously a lot, and based on the model a large portion of the equipment would reach absurd temperatures. Furthermore, in the model, convective processes are neglected so the actual necessary energy could even be a factor of order 1 larger or smaller.
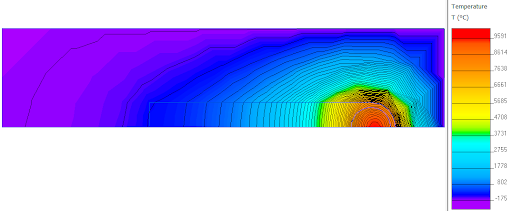
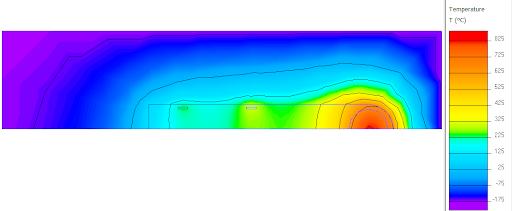
To overcome this, a very small, long heat source along the length of the digger is proposed. In this case, the same water layer can be established, but with only 20% the total power: 10 kW for both sources. Mind you that this power source is a hollow cylinder.
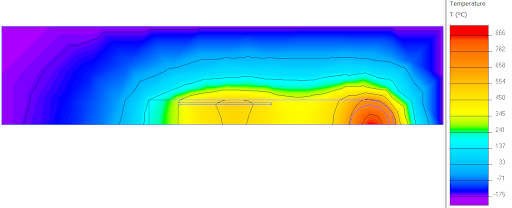
The last configuration is one in which the cylinder has been replaced by two rings that produce 4 kW each.
Furthermore, from the power output of the source, the maximum speed of the digger can be determined. This is based on the amount of ice in front of the digger that it can melt per second. The energy H required per kilogram of ice to melt it is equal to: H = lf+Cv*ΔT, where lf is the latent heat of fusion, the energy required to induce a phase change, Cv the heat capacity and ΔT the temperature difference to be established. for ice, lf=334kJ/kg, Cv=2.05kJ/kg/K and in the case of Europa ΔT=50 -- 200 = 250K at least. The lower temperature boundary is the lowest temperature we expect to encounter on Europa, whereas the upper temperature boundary is there to ensure that the water stays molten for a while. This temperature difference will likely decrease as the digger gets deeper into the crust, because the ice warms up slowly. However, in the worst case this will allow the digger to go somewhat faster. The total energy then becomes H=334+2.05*250=846.5kJ/kg. The energy per unit volume can be determined from the density of ice: 920 kg/m3, resulting in 778780 kJ/m3. The digger’s speed v can be calculated by dividing the total power used to melt the ice directly in front of it P by the energy required per unit volume H and the total frontal area of the digger A. The latter is equal to π*r2, where r is the radius of the digger's cylindrical part. v = p/(H*π*r2) For instance, at 10 kW and a radius of 25 cm, the velocity of the digger becomes 65 μm/s. At this velocity, the digger would take about 4.8 years to dig 10 km. At 1 MW effective power, this is reduced to a much more manageable 17.7 days.
Scaling up the power source
Considering the amount of power required for the digger, accounting for losses is a luxury not available to us. Hence, the heat production should be done as close to the place where it is needed, namely in front of the digger. An additional advantage of this is that it will weigh the digger down, meaning it may push itself through the ice a little faster. RTG source selection (If not mentioned otherwise, radioisotope data in the remainder of this section is derived from this document.) There are several consideration to be made when selecting a power source for an RTG:
- Sufficient (power) density
- Sufficient duration
- Right type of radiation
- Non-critical
(Power) density
In particular in space missions, the power density of the source is important. For instance, it is possible to get a block of Am241O2 that produces 10 kW, but at a power density of .097 thermal Watts per gram, this block would weigh 103 kg. As a comparison, Am2442O2 has a power density of 2.01 Wh/g, resulting in 10 kW of power at only 4.98 kg. Furthermore, the material's density itself matters as well. To illustrate: Cs137Cl has a power density similar to Am241O2, but not 1/3 the density. As a result, a block of Cesium Chloride must be 3 times as large to produce the same power as a block of Americium Dioxide, whereas both blocks have about the same mass. Thus, the perfect power source should have a large enough power density to decrease its mass, as well as a high enough mass density to decrease its volume. The mass of the power source is equal to the desired power divided by the power density. This mass should be divided by the mass density to find the volume of the power source.
Duration
One disadvantage of RTGs is that due to the decay of the radioactive source, the power of the source slowly decreases over time. An isotope with a sufficient half-life should thus be chosen accordingly. Consider 3 isotopes:
- Uranium-235, with a half-life of 8.9*10^8 years Uranium half-life
- Plutonium-238, with a half-life of 87.74 years
- Cerium-144, half-life of 284.4 days.
The activity of isotopes is proportional to the number of isotopes. In particular: A(t) = A(0)*2(-t/t(1/2)) with A(0) the initial activity (to which power production is proportional) and t(1/2) the half-life of the radioactive source. Filling in the half-lives for t = 7 years, a rough estimate of the travel time to Europa, results in activities at the time of arrival of 1, 0.946 and 0.00198 times the initial activity for U-235, Pu-238 and Ce-144 respectively. This goes to show that an isotope like Cesium-144 would reduce in power by 99.8% over the course of the journey to Europa. To achieve a power of 10 kW upon arrival, the initial power source must then be 5 MW. This power requires a 200 kg Ce source. Uranium and Plutonium, on the other hand, retain most of their activity over the course of the mission. However, this would imply that Uranium would be a better source than Plutonium. This is not the case. Even though the activity of U decreases more slowly than Pu, the activity of Pu is higher than U. This can be understood by realising that U has a longer half-life ‘’because’’ it decays slowly. If it decayed faster, all the atoms would deplete in a much shorter timespan. There is a general inverse correlation between activity and half-life. As a result, the half-life of the perfect isotope is too high nor too low, preferably about an order of magnitude greater than the duration of the mission. The power density upon leaving earth can be calculated as follows: P(t) = P(0)*2(-t/t(1/2)).
Type of radiation
Now we shall consider the type of radioactive decay that the perfect radioactive source undergoes. To avoid radioactive contamination of environments or the destruction of on-board equipment, it is important to shield the radioactive source. At the same time, due to weight considerations, it is also important that said shielding is not too thick. To achieve the latter, the right type of radiation has to be chosen. Of the four main types of decay - alpha, beta, gamma and neutron - alpha is by far the most easy to mitigate, with air half-value layers of the order of cms. Hence, it also makes sense to choose a radioactive source that decays via alpha radiation. Examples are Plutonium-238 and Curium-244.
Non-critical
Lastly it is important that the mass of the radioactive isotope does not exceed its critical mass. If a hunk of the isotope exceeds the critical mass, the decay of atoms in the isotope will accelerate the decay of other atoms in the isotope, causing a runaway chain reaction like the ones that occurred in Tsjernobyl and Fukushima, like the ones that are induced in nuclear bombs. If the mass is kept small enough, or the geometry is chosen efficiently, the atoms don’t interact with each other as much and the chain reaction will remain under control. These comparisons hopefully suffice to illustrate why a runaway chain reaction is undesirable. The considerations to be made are as follows:
- ’’High critical mass of the isotope’’: This allows for a larger amount of isotope to be taken along on the mission.
- ’’Geometry’’: As in a spherical configuration the atoms are most densely packed together, the critical mass is lower in a sphere. Critical masses
The best power source
Below is presented an overview of the most promising radioactive sources, with from left to right their half-life, power density, mass density, critical mass, P0, mass, volume, attenuation layer. To determine what source is ‘most promising’, the right principal type of radiation and the right half life of some isotopes were picked from the article on RTG source selection mentioned in the beginning of this section. Most information was gathered from that article as well. To determine the attenuation layer, the lead layer thickness required to reduce the gamma dose (alpha does not transmit through lead at all) at 1 m to .1 mSv. In this article (Curium attenuation), the shield required to meet precisely that condition is given for Curium with lead. For other sources this information appears impossible to find. Thus, to get an order of magnitude estimation of the other materials, we will be using the ratio between this value and the values listed in the article on RTG power sources. With an attenuation thickness for Cm-244, this ratio is 2.155. Results are in the table. Curium mc Plutonium mc Americium mc and density The isotopes chosen were Curium-244, Plutonium-238 and Americium-241.
| t(1/2) (a) | ρ (kg/L) | ρw (W/kg) | mc (kg) | P(0) (W) | m (kg) | V (L) | r (dm) | d (dm) | ml (kg) | |
|---|---|---|---|---|---|---|---|---|---|---|
| Cm | 18.11 | 13.51 | 2840 | 20.07 | 13072 | 4.6 | 0.341 | .433 | 1.1 | 167.4 |
| Pu | 87.74 | 19.33 | 560 | 8.2 | 10568 | 18.9 | 0.976 | .615 | 0.0547 | 3.224 |
| Am | 432.0 | 13.66 | 110 | 56.4 | 10113 | 91.93 | 6.73 | 1.17 | .3832 | 102.1 |
The two most important conclusions to be drawn from the table are the following: Cm, at the required mass, has less than a quarter of its critical mass. Pu and Am have approximately 2 times the mass. So, Cm can be used as a solid sphere, whereas for Pu and Am, a different geometry would have to be chosen, or multiple smaller spheres have to be used. Furthermore, disregarding the critical masses of the isotopes, Cm would require a sphere with a radius of 15.3 cm, weighing 172 kg; Pu a sphere of radius 6.7 cm, weighing 22.1 kg; and Am radius 15.5 cm, weighing 194 kg. Considering the critical and required mass of Americium, it can be quickly dismissed as a useful source. Thus, to choose between either Cm or Pu, we will attempt to make different suggestions for the geometry of the Plutonium, and determine whether the different geometries still result in lower masses than Curium. The first is to split the single plutonium core into 3 separate cores. The mass of these will be the same, but each will now have a radius of 4.27 cm. To coat these in a layer of .547 cm of lead, requires 1.6 kg each, or a total of 4.8 kg, bringing the total mass up to 23.7 kg. However, this configuration does not lend itself for a very homogeneous temperature distribution. For that, we will use a hollow hemisphere with a thickness of half the critical radius (rc=4.66 cm). To obtain the required mass of 18.9 kg, this sphere should have an inner radius of 6.97 cm. To coat this hemisphere with the required layer on both in- and outside (the outside for protection of the environment, and the inside for protection against itself), requires 10.0 kg of lead, for a total of 28.9 kg. The last suggested configuration is a torus with a square cross section with a diagonal cross section equal to the critical radius as listed here (4.04 cm). The major radius of this torus is then 9.52 cm. To cover this torus in lead, requires 6.8 kg of lead, bringing the total mass to 25.7 kg. Each of these configurations has its own benefits and disbenefits. The 3 spheres probably don’t create a very homogeneous temperature distribution, which may cause the digger to lose efficiency when digging, or have trouble going straight. The hollow sphere is the heaviest of the 3 configurations, and the torus fails to heat the front of the digger, where the heat is needed most. The best solution can be found in a combination. The idea is to create a sphere of plutonium with a torus around it. The sphere and torus are separated by a double layer of lead, then the radii are varied to get the exact right mass of plutonium. The cross section of the configuration is shown in the figure below:
Calculations give a radius of the sphere of 4.47 cm, still below the critical radius, and a major radius of the torus of 7.04 cm. The total mass of shielding is 6.4 kg, resulting in a total of 25.3 kg. This is still a bit above the mass of the spheres, but a little below the mass of the single torus. The configuration is radially symmetric, and due to the smaller torus radius can be placed higher up in the digger.
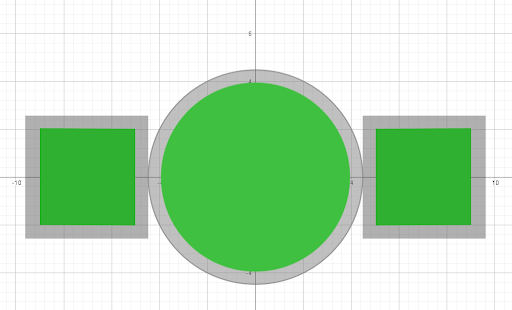
Furthermore, if deemed necessary for sufficient heat transfer, the sphere can be pushed up a little further than the torus as well. This way the Plutonium-238 does not reach critical masses, and the total mass is still well below the mass of the total Curium-244 source.
In conclusion, the best power source for the digger is Plutonium-238, due to its high alpha ray energy density. The configuration of source material best suited for our purposes is the sphere-torus combination.
The design of the spacecraft
The lander that we envision consists of three different parts that, at first, land on the planet as one vehicle. This lander then splits off a digger vehicle (not sure where this digger vehicle will be on the lander yet), which makes its way through the solid ice. The lander itself will remain on the surface, whilst physically remaining connected to the digger. This physical connection consists of a wire (fiberglass or electrical) that runs between the digger and the lander. This physical link was chosen because of the ice on Europa. This ice will most likely freeze shut any tunnel that the digger makes, which makes a wireless connection through the ice extremely unusable. This lander is furthermore equipped with a few tools to perform some experiments on the surface. These tools consist mainly of the same tools that the digger has on board, namely a seismometer, a spectrometer, a camera, and a tool to perform gas emission experiments.
When the digger reaches the underside of the ice and is about to enter the water, the digger locks itself into the bottom of the ice. Inside this digger is a compartment that houses an AUV. This compartment will open to let the AUV into the water. The digger itself will remain stationary in the ice and communicate through sound waves with the AUV. The AUV, then, will start exploring the subsurface ocean as a submarine, if you will. During this exploration, this submarine will take pictures and perform spectrometric experiments and gas emission experiments.
Communication between the lander and Earth
On Earth, NASA uses a system called DSN to communicate with spacecraft NASA DSN. DSN stands for Deep Space Network, and it allows NASA to communicate with spacecraft as far as Opportunity 1, which is, by now, outside of our solar system. This means that a satellite around Europa is not absolutely necessary, but it may provide a faster way to get our data to Earth. Since Europa is tidally locked to Jupiter (as written here), and since its orbital period is about 3.5 earth days (as stated here), a large part of the time, the lander will not be able to directly send data back to Earth. This is due to either Jupiter being in the way, or Europa itself is in the way when the lander is at the back of Europa, relative to Earth. If, for this mission, there was another satellite orbiting Europa or Jupiter, the lander would be able to send its data to this satellite with a much higher data rate. During the Mars Curiosity mission, NASA has an orbiter around Mars, which intermittently communicates with the Rover. The communications between Curiosity and this orbiter are about 150 times as fast as the communications would be between Curiosity and Earth directly. Since this orbiter has more power that it can focus on communications, the data will reach Earth much faster through this orbiter, than it would directly to Earth.Communications of Curiosity.
Necessary bandwidth
Of all the instruments that our Europa lander is expected to carry, the camera is expected to generate the most data. During the Mars Exploration Program, which has already lasted for over a decade, the camera of Opportunity would generate an expected 97.36 Gigabytes of data (as budgeted here). Since our mission is not expected to last nearly as long, the expected bandwidth necessary for this mission is also not expected to be particularly large. If our mission is expected to only last for a fraction of the time that the Mars Exploration Program has already lasted, an orbiter may not even be necessary to obtain all data generated on Europa within reasonable time.
Wireless communication through ice
Ice is quite a good isolation material when it comes to wireless signals. Radio signals are very quickly absorbed by ice (this study found), and optical signals do not travel through ice very far either. Low frequency radio waves, however, are a medium that does travel through ice better (as written here). The question arises, however, whether these radio waves will survive through 10 km of ice, as that would be a very unusual situation here on Earth for researchers to test on. So we assume wireless communication through the ice crust on Europa is for all intents and purposes impossible.
Electrical cable as alternative to fiberglass
In current society, most electrical cables are made of copper or aluminum. These cables are used for their high conductivity and low resistance. Where aluminum is mostly used for heavy industrial wirings, copper is at the core of most everyday electrical cables. Copper’s conductivity decreases as temperature increases, but as the temperature goes down, the conductivity increases. This happens until about 30 Kelvin,at which point lower temperatures drastically decrease copper’s conductivity. Even though copper’s conductivity at around 30 Kelvin reaches a very high point, at no point is copper itself considered to be a superconductor.

Where will the experiments be performed?
Both the initial lander, as well as the Autonomous Underwater Vehicle (AUV) possess instruments that will be used to perform experiments. The initial lander features one more instrument than the AUV does, and the lander will remain stationary on the surface. Here, the lander will perform the spectrometric experiments on the ice of the surface, as well as gas emission experiments. Furthermore, the lander will deploy its seismic sensor on the ice to perform seismic readings, as well as use its camera to take pictures of its surroundings.
The AUV will make its way into the subsurface ocean in order to make valuable use of its sensors. Save for the seismic sensor, the AUV is equipped with the same tools as the lander. The AUV will perform spectrometric observations on samples of water, as well as any soil, if there is anything of the like present. The AUV will also perform gas emission experiments on samples of the same material (e.g. water at different depths and soil, if available). Since it wouldn’t make sense for the AUV to perform seismic experiments in the water, the only other thing that the AUV will be doing is taking photos from underwater (and hopefully capture some life on them).
Movement under water
To be able to manoeuver under water, the AUV needs to have a propulsion system. The simplest propulsion system that would (probably) work, is one (or more) propellers, like the ones that submarines have. Underwater drones are already available to the public, and most of them seem to feature this style of propulsion.
Another alternative propulsion method may be one that is designed by means of biomimicry. One example of such a project can be seen to the right (NOA Marine). In this image, the drone mimics the movements of a squid to propel itself forth. Besides this design, there have been multiple other designs that rely on biomimicry, for example mimicking a manta ray.
Sluiswerking op extreme dieptes is helaas niks over te vinden
What to do with extraterrestrial life (updated)?
It is, of course, not impossible that a mission to Europa will find life of some form or another. We will then wish to learn from this life, but the ways in which we can ethically allow ourselves to do so may be limited. The question is: How limited? Should we find extraterrestrial life, how does this impact the mission? To answer this question, it is important to first consider the moral value of extraterrestrial life in itself. The following dissertation outlines why all life, terrestrial or not, should be considered to have some intrinsic value. We adopt this view, acknowledging that at the same time extraterrestrial life has extrinsic value as well, as it can serve to further scientific research (as argued in this article). Furthermore, one should note that, if something does not have intrinsic value, this does not mean it should not be preserved. If something has value to something with moral value, it acquires value. So it also goes for the Europan environment, which is important for the life present on it, as well as for our own research purposes.
We will discuss some different forms of life that we might encounter during the mission and how we should handle in each individual case. First of all, sentient versus non-sentient life. If we find life at all, it is far more likely that it will be microbes or other non-intelligent alien life than sentient otherworldly beings (as argued here). As stated in previous paragraph, all life should be considered to possess intrinsic value, which means that we would treat the potential sentient and non-sentient life the same way. In the (previous/following) section experiments, one can read the experiments that we would like to examine on Europa. None of the experiments will harm or disturb the potential life directly. But the life can be harmed indirectly too, which we will explain later on this section. Second of all, carbon-based life versus non carbon based life. On earth, all known living things are based on the element carbon. But scientists belief that there could be an alternative chemical basis for life, for example silicon-based life (according to this article). There could be creatures in unimaginable forms and capabilities on Europa, but since it is also “life” we will treat these potential creatures the same as carbon-based life, with the assumption that they have some sort of intrinsic value.
Another thing that needs to be excluded, is the possibility of contamination and thereby affecting Europa’s environment or ecosystem. This is the indirect harm of life on Europa as addressed before. Contamination can be done in different ways; via human bacteria or viruses that end up on the robot during the construction of it, wear or rust of the metals in the robot or a radioactive source that poisons the environment. The first possible way of contamination, human bacteria, seems to be no problem at all. According to the following source, bacteria die slowly when they are exposed to freezing temperatures. And since the robot is traveling through the space for about 6 years in -270 degrees of Celsius, we can assume that all possible human bacteria are dead when the robot arrives on Europa. Viruses are a higher risk for contamination. There are multiple researches done to the surviving of viruses in spatially conditions, and some experiments proved that viruses can actually survive in these conditions for a long time. The longest time a virus was exposed to (and survived) the space environment was 2 years. Since there is no data of the decline of this specific virus, we can not make a prediction if this virus will survive the 6 travel years to Europa. And thereby we can not exclude that some viruses might be transferred from earth to Europa during the mission. The only thing we can do is make sure that the robot goes into space as virus-free as possible (as can be read here). There is also a possibility that the robot could catch a virus on its way to Europa. But to date, almost no research has looked into the possibility of viruses “living” in space. So until this remains unknown, we don’t know if we have to take this risk into account.
For the last two ways of contamination, wear or rust of the metals in the robot or a radioactive source that poisons the environment, the choice for materials and energy source should take these dangers into account. So the materials should be the best non-wearing and non-rusting materials available. And we must look for an energy source that does not have radioactive leakage, so the environment of Europa won’t be in danger. We will discuss this later on.
Structure
- Introduction - general knowledge about Europa (week 1)
- Physical consideration about the presence of water on Europa (week 4)
- Presence of a sub-surface ocean (week 4)
- Indications for life
- Problem statement (partly week 1)
- Users (week 3)
- User requirements (week 4)
- User preferences (week 4)
- Constraints (week 4)
- Measurability requirements and constraints
- On the ice or in the water? Examine Europan core - section 3.2 (week 3)
- Experiments
- Which experiments (week 4)
- Where to execute (week 5)
- General
- Communication
- Earth to surface receiver (partly week 5)
- Via satellite?
- Necessary bandwidth
- Surface receiver to sub-surface receiver (week 4)
- Fiber options
- Electric cable options (week 5)
- Wireless communications through ice
- Communications under water (week 4)
- Wireless communication
- Earth to surface receiver (partly week 5)
- Autonomous
- What to do with extraterrestrial life (week 5)
- Sentient vs non-sentient life
- Carbon-based vs non-carbon-based life
- Possibility of contamination
- How long does the mission need to last (week 5)
- Communication
- From Earth to Europa
- General description about the journey and the risks
- Landing and surviving on the surface
- Where to land (week 4)
- Tectonic activity (week 2)
- The effect of temperature and pressure on the functioning of the robot (week 2)
- Shape and function of the lander
- Executing experiments
- Through the ice - from surface to the sub-surface ocean
- Power
- Power source in digger or on surface (week 5)
- Estimating how much power is needed (week 5)
- The most suited power source (week 5)
- Functioning of an RTG on Europa (week 2)
- Where to place a sub-crust base (week 4)
- Modelling temperature on the digger (week 4)
- Shape and function of the digger and sub-crust base
- Executing the experiments
- Power
- In the water
- Movement submarine (week 5)
- Is it possible to go to the seafloor (week 4)
- Shape and function of the submarine (partly week 5)
- Executing the experiments
- Design (partly week 5)
- Submarine
- Sub-surface crust
- Ice digger
- Lander
- Discussion
- Planning